Guest commentary by Martin Vermeer
On December 7, 2009 the embargo expired, and my and Stefan’s joint paper ‘Global sea level linked to global temperature’ appeared in the Proceedings of the U.S. National Academy of Sciences. It had been a long time coming! But this post is not so much about the science as about the process, and about how a geodesist from Helsinki and an oceanographer from Potsdam, who to this day have never even met, came to write, to the surprise of both of us, a joint paper on sea level rise.
My own entry into climatology happened only a few years ago. A significant trigger was RealClimate, which I had learned to appreciate as one of the rare reliable Internet sources amidst the junk. Contributing to the oft-slandered science is my small ‘thank you’ and revenge as a scientist.
As I remember, it was the commenter calling himself Rod B. who enquired, sometime August 2008, what the story really was with Rahmstorf (2007). Trying to answer, I ended up reading the paper and getting interested. What seduced me was the simplicity of this, so-called semi-empirical approach: linear regression of sea level rise dH/dt against temperature T, yielding two unknown parameters: a regression coefficient a, and an intercept, or ‘equilibrium temperature’, T0. See our Ups and downs of sea level projections for a more detailed explanation.
The curve of temperature as a function of time over the 20th century has three parts: a steep rise in the beginning, a flat middle part commonly attributed to aerosols, and a very steep upswing at the end. Physically one would expect for the curve of the sea level rise rate dH/dt as a function of time to look rather similar, as indeed it does: this justifies the Rahmstorf (2007) approach of regressing the one against the other. Looking more carefully however one sees that the dH/dt curve has slightly more of an S-like shape, turning downward in the middle, before swinging up again at the end.
This suggested to me that, in addition to a proportionality to temperature T, sea level rise would also contain a term proportional to the time derivative of temperature, dT/dt. In other words, global sea level would be a good global thermometer, but with a ‘quirk’. I could even think of a physical mechanism for such behaviour.
I contacted Dr. Rahmstorf, proposing the idea: one would expect the ocean surface to warm up rapidly to completion, contrary to the deep ocean and the continental ice sheets. This would argue for a term, in addition to the secular a (T – T0) term, of form b dT/dt. Stefan’s response was cautious; not surprising, as being something of a media figure in Germany surely means that he has to contend with his share of cranks. But he suggested I look myself into the idea, which I subsequently did: in for a penny, in for a pound.
I downloaded Stefan’s script, modified it, did the first computations with the same real tide gauge and temperature data Stefan had used — surprise: negative b. Hmmm, strange. That was for real data from the real Earth; what would happen if I applied the extended relationship to simulated data from the same general circulation model (actually, an Earth system model) for the period 1900-2100 that Stefan had used in his paper for testing his relationship? This model was in one essential way very much simpler than reality: it completely lacked the contribution of land ice melting to sea level.
Stefan helpfully sent me Matlab snippets and model output, and indeed I got it all working. What was more, the disagreement found by Stefan for the late 21st Century — between sea level rise as predicted directly by the model, and indirectly through the semi-empirical relationship between temperature and sea level rise — went almost completely away when using the new, extended relationship. With a positive value for b, just as expected from theory for an ocean surface water response.
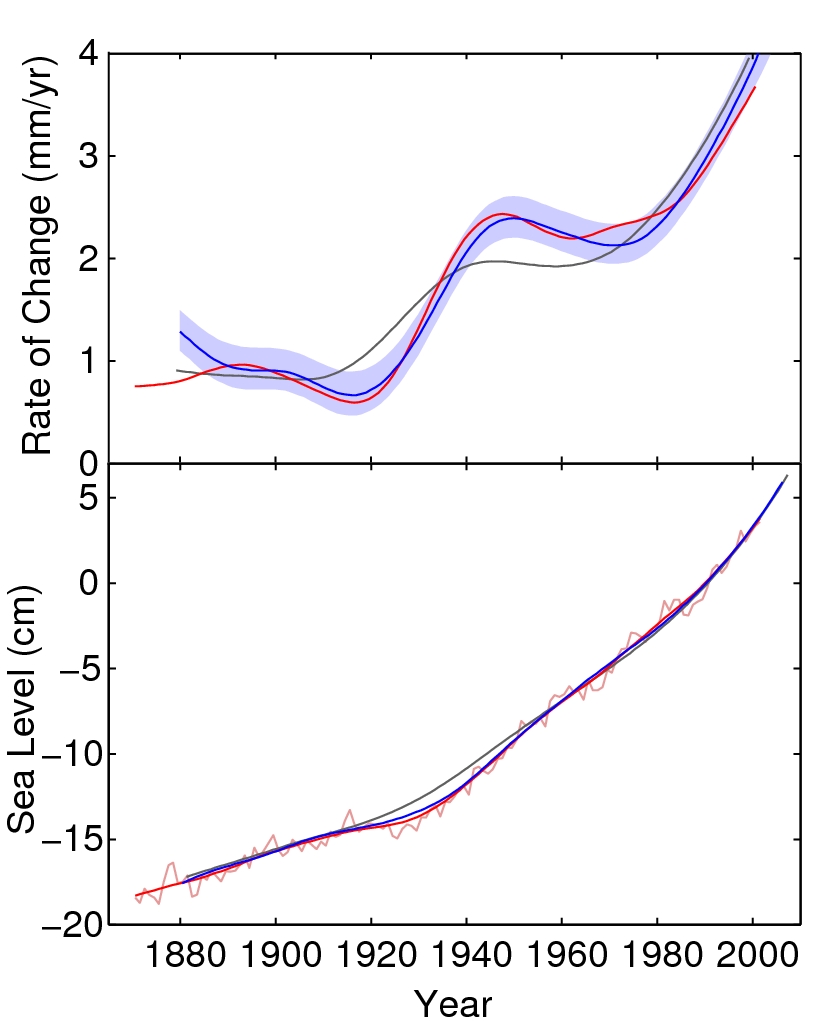 |
| Global sea level against time. Top, sea level rise, bottom, sea level itself. Red, sea level from observations; blue, with uncertainty band, the fit from global temperatures using our new relationship; black, the fit using Stefan’s original relationship. The thin red wiggly curve shows annual sea level values. |
That was encouraging, but what again about the real data? Remember that this is real observational data from tide gauges, altimetric satellites and meteorological stations, warts and all, with a very imperfect spatial sampling both for the tide gauge data and for the surface temperature data. Nothing like the clean, formally perfect model output of truly global mean surface temperature and sea level.
At that point I was about to give up.
I remembered however Stefan mentioning a ‘reservoir correction’ and decided to see if that made a difference. It was not hard to find Chao et al. (2008), who had painstakingly compiled a list of all man-made reservoirs the world over, and the amount of water stored in them. I fitted a simple arctan function through their water storage curve and added that to Stefan’s already extended script. All that water, up to 30 mm sea level equivalent, that should have been in the ocean was progressively kept bottled up on land as dams were being built: a known correction that should be applied.
Wow. Introducing the b term had already improved the Pearson correlation r of fit from 90% for Stefan’s original relationship to 97%; nice, but hardly on its own compelling. Bringing in the Chao et al. man-made reservoir correction brought it up to 99.2%!
Slowly it dawned upon me that, hey, maybe I’m on to something real here, something based in physics: it seems the world ocean can be a remarkably good global thermometer, once you get to know its quirks.
 |
The world ocean, a pretty good global thermometer (drawn using GMT). |
Stefan relates the moment when he realized that I had something worth publishing: January 16, when he saw the results of the ‘millennium run’ that I had done on the data he had sent me. All of the volcanic explosions over the last thousand years, which were translated first into top-of-atmosphere radiative forcing and then turned into sea water thermal contraction and a drop in modeled sea level, were faithfully reproduced in the sea levels obtained from the model temperatures by my new relationship! A beautiful performance on what are large, rapid and erratically occurring excursions in both global temperature and sea level. And that’s how Stefan came on board.
With the small number of independent data points we needed to make sure we were not ‘fitting an elephant‘, so I read up on statistics during winter 2008/2009, and in particular, information theoretical methods like the Akaike Information Criterion. The model intercomparison was useful for just that. I’m not the only one studying these ideas, and I learnt a lot from tamino and James Annan’s Empty Blog. Jaynes (2003) was also on my 2009 Christmas reading list; Hypothesis testing, null and alternative hypotheses, confidence bounds and all that, is a traditional approach to statistics that is easily misunderstood and often misused. Statistical refutations of “silly null” hypotheses abound — like the silly null of no relationship between temperature and sea level rise. If this sounds all cryptic to you, I don’t blame you. Pick up Jaynes (2003), it’s an eye-opener.
As part of his contribution, Stefan tightened up the draft paper to be suitable for submission to Nature. Nature gave us some very helpful reviews which we used to further improve our manuscript. The most useful reviewer remark had to do with the extraction of water from underground aquifers, a process potentially almost as important as the artificial reservoir storage that we did take into account — only, nowhere in the literature was there an equally painstaking accounting exercise to be found as what Ben Chao and colleagues did for the reservoirs. So, we settled for a sensitivity analysis, skillfully whipped up by Stefan.
Nature turned us down, like they do over 90% of manuscripts; had they accepted, the paper would have been out already in summer. We resubmitted to PNAS who obtained three further helpful reviews, the paper was improved yet again and finally published in December. As it happens, this landed it right on top of the Copenhagen meeting.
Stefan tells me that we have exchanged over a thousand emails in the run-up to this paper. I see some poetry in that number being close to that of the East Anglia stolen email selection. Easy, informal email plays a vital role in the work of climatologists, and the loss of trust in its confidentiality could be very disruptive for the science: if the internal discussions of an authoring team would have to be expressed with the same care as the finished product, not a lot of authoring would get done.
Would I have dared, or managed successfully, to submit to a top journal all on my own? Hardly. It is an illusion to think that you can just enter a field that’s not your own and become a productive researcher, whatever you might read or what denialists-of-service may pretend. There is a lot of domain knowledge involved, and precious little of it is simple. In this case, I did learn a lot (and I continue to do so), but this takes both a willingness to learn, and great teachers. RealClimate, and the community it represents, are an indispensable resource for that.
Still waiting for Al Gore’s cheque…
P.s. Over at Nature Stefan has a commentary on sea level today.
References
Martin Vermeer and Stefan Rahmstorf (2009): Global sea level linked to global temperature, Proceedings Nat. Acad. Sci. 2009 vol 106 no. 51 pp. 21527-21532, DOI: 10.1073/pnas.0907765106, open access link
Jonathan Overpeck and Jeremy L. Weiss (2009): Projections of future sea level becoming more dire, Proceedings Nat. Acad. Sci. 2009 vol. 106 no. 51, pp. 21461-21462, DOI: 10.1073/pnas.0912878107 link.
Stefan Rahmstorf (2007): A Semi-Empirical Approach to Projecting Future Sea-Level Rise, Science 315, 368-370, DOI: 10.1126/science.1135456 link
B.F. Chao, Y.H. Wu and Y.S. Li (2008): Impact of Artificial Reservoir Water Impoundment on Global Sea Level, Science, 320, 212-214 link
Edwin Jaynes (2003): Probability theory: the logic of science. Cambridge University Press, ISBN 0-521-59271-2.
Re rising tides.
Tectonic plates move quite rapidly when the globe is cooling, You may witness many earth quakes also because of the shrinking effects of the Earth and the effects of the oceans cooling after 1998 as their related structural movement. Australia and the Antarctic plates are rising and slowly moving north.
Hence there are may future examples of these happenings in the past when Earth had Little Ice Age period, there were over 90 eruptions, and 4 in 1680 alone, and we have entered into another cooling scale of this same period, beginning around 1998. I noted in the original paper that the graphic stops at 1990 odd. Strange that in 2010, this did not show the decline that has happened.
The history of Earth is cyclic and found to have the same qualities that have already been expressed by many of the readers of your promotion.
Gilles, Hank,
This is a strawman argument. The only way to stop using fossil fuels is through the judicious application of thermonuclear weapons on the world’s population centers and critical infrastructure. No one is seriously advocating this.
What’s been advocated is:
1) to burn through the fossil fuels reserves (much) more slowly
2) CO2 sequestration
Gilles has provided no argument against 1) or 2). Even modest efforts would mitigate ocean acidification, GW and SLR somewhat. Additionally, 1) would preserve the reserves for later use (for the purpose of adaptating to long-term SLR for instance). What would be the downside exactly, Gilles?
Hank (88), on Gilles,
The definition of a fanatic is “someone who can’t change his mind, and won’t change the subject.”
Interesting !
But I have reservations about the limitations of the methodology.
Given the dynamics of plate tectonics, the movement of sediments to the oceans by the worlds rivers,Coastal erosion, none of which have been delt with in the paper; how does one determine mean sealevel on a planetary basis with any reliability.
Sea level rise will combine factors for thermal expansion and icecap melt and plate tectonic movement. A warming of the icecaps with enhanced melting will result in reduced volumes of seawater reaching maximum density and sinking to maintain the superhaline currents, thus slowing the current rather than increasing the deep ocean temperature.
one of the more intractible problems is the variance of surface sealevel monitoring, in areas of stable plate areas mony monitoring stations show little sealevel rise in the last 100 years, on th other hand a guage on the south of Haiti is now 7 ft out of water while on the north its 7 ft under water. Mountains are being ground down at about thesame rate they are being pushed up, but i’m not aware of any studies on the impact of sedimentation on men sea level.
We still have a long way to go before we can predict with any certainty into the future.
“The history of Earth is cyclic and found to have the same qualities that have already been expressed by many of the readers of your promotion.”
Only when you postprocess the record and state that there is a median.
Once that is done, you can look at each time it was higher than that followed by at SOME point a time it was lower than that and say “cyclic”.
The sun’s output is cyclic.
It starts off frigid and dark, lights up hotter and then cools down to frigid and dark.
Cyclic.
Or maybe using “cyclic” like that (as you have done TS) is complete bollocks.
Oops, that should have been gilles.
Waqidi Falicoff #66: Maunder minimum? Tell your buddy to check out the latest satellite AMSU-A data as explained at my blog.
As to your direct question, why is it reasonable (as in the blog you refer to) to set dH/dt to a constant? Note that the author’s logic requires a temperature trend growing as exp(-at/b). This is a growth rate that rapidly converges to zero, unless you reverse the sign by making one of the multiplied constants (a or b) negative. Big surprise: it results in constant sea level rise when you plug it into the equation given in the paper covered here. Oh and the author fibs slightly about using the same constants as in the paper in his own calculations. In the paper, b = 2.5
101 AC :”What’s been advocated is:
1) to burn through the fossil fuels reserves (much) more slowly
2) CO2 sequestration
Gilles has provided no argument against 1) or 2). Even modest efforts would mitigate ocean acidification, GW and SLR somewhat. Additionally, 1) would preserve the reserves for later use (for the purpose of adaptating to long-term SLR for instance). What would be the downside exactly, Gilles?”
Well, I haven’t provided any argument against that simply because it hasn’t been discussed in this thread up to now ;). But since you mention it now, I would answer
1) I don’t think that burning more slowly the same amount of CO2 will change anything in the long term asymptotic state of the Earth – and the problem is precisely that Stefan and Martin’s theory ASSUMES that sea level rise is a slow change with a very long characteristic time of several centuries. So roughly speaking the final state depends on what has been burnt within this relaxation time, and not on the details of how it has been burnt within it.
2) CO2 sequestration is technically possible, but it has a cost. But saving fossil fuels is interesting only because they are used to produce wealth. So in some sense, CO2 sequestration goes against saving – it is a waste of energy actually. So again one should carefully investigate if the marginal cost of CO2 sequestration is justified by its benefit. Note that as the externalities of CO2 are CUMULATIVE, it means that the cost increases with the total amount burnt since the beginning of industrial civilization, whereas the benefit is almost constant for a given level of the technique and the economy (which can improve with time of course). It means that the answer is probably that CO2 sequestration becomes interesting above some level of cumulative amount of burnt fossil fuel. Same issue again : which level?
Anonymouos Coward@101,
Let us know as soon as anyone comes up with a viable carbon sequestration scheme.
“Well, I haven’t provided any argument against that simply because it hasn’t been discussed in this thread up to now”
It has several times.
EVERY SINGLE TIME “use less fossil fuel” comes up, YOU say “can’t be done” or “that would pauper the third world” or “that would ruin the first world economy”.
But revisionism is the soul of denial.
“Given the dynamics of plate tectonics, the movement of sediments to the oceans by the worlds rivers,Coastal erosion, none of which have been delt with in the paper; how does one determine mean sealevel on a planetary basis with any reliability.”
The same way, despite the dynamics of cell growth, muscle relaxation and posture, you can determine how tall someone is quite reliably.
I.e. those elements are bounded changes.
> I don’t think burning more slowly the same amount of
> CO2 will change anything in the long run
You don’t think about the rate of change.
You’ve ignored all attempts to point you to the science.
Rate of change is the fundamental, most important factor.
Gavin, would I be out of line if I just start posting “Oh shut up” in replies?
CFU :”EVERY SINGLE TIME “use less fossil fuel” comes up, YOU say “can’t be done” or “that would pauper the third world” or “that would ruin the first world economy”.”
I think you’re slightly OT. The point I raised here was only that if the model of sea level rise with a dL/dt = a(T-To) term is true along the century , it means that there is a very long (1000 yrs or so) relaxation time and that the rise will continue anyway for centuries even if we stop all the warming just now, leading to a several meters rise whatever we do. So saying that “we should do that or that to limit the rise below 1 m after 2100 ” is just an already lost bet : there is nothing that can prevent the rise to carry on at an almost constant rate for centuries. This is just simple maths and I don’t think it has been seriously contested even by the authors of the theory
Now if OT is allowed, when I say that limiting use of FF will cause more people to be poor, I’m not the only one to say that. For instance saying ” The only way to stop using fossil fuels is through the judicious application of thermonuclear weapons on the world’s population centers and critical infrastructure. No one is seriously advocating this.” implicitely means that we NEED FF – else why should it be a problem to stop their use? and if we need them, then limiting their total amount means that we accept to limit the access to what they are needed for.
I can accept the theoretical statement that this limitation could be justified in regard to the drawbacks they would produce. But saying “nobody advocates their immediate cut-off” means that this point is not yet reached up to now – just now, it seems still more interesting to burn at least part of them than nothing at all. So my question is very simple : above which level won’t it be the case anymore, and why exactly ? if you can’t offer a precise answer and justification to this, don’t be surprised that many people in the world will rebel against this injunction.
“Gavin, would I be out of line if I just start posting “Oh shut up” in replies?
”
Gavin can respond for himself, but I think that you would be simply useless.
Gilles (#108),
The ultimate effect of fossil fuels emissions are dependent on the time profile of the emissions because there are (very) slow temperature and CO2 concentration feedbacks involved. Lower emissions for a longer time would result in lower CO2 and methane peak concentrations, lower peak temperatures and so on. Please read up on how the known slow feedbacks (albedo, carbon cycle) work.
Slower emissions would allow for more carbon sequestration, which needs not be very expensive as it should be possible to leverage processes such as photosynthesis or the Urey reaction.
My understanding is also that it is unlikely that several meters of SLR could be avoided merely by cutting emissions. But it does not follow, as you seem to be implying, that failing to cut emissions would have no consequences.
Your economic considerations regarding the use of fossil fuels are too abstract. Some uses are currently essential and people derive great benefit (as in staying alive) from them. There are good substitutes for other uses and some uses are simply frivolous. Greater value per mole is derived from the use of small amounts of fossil fuels than larger amounts, as the relationship between the quantity supplied and the price of the fuels demonstrates.
The desirable level of atmospheric CO2 is more relevant than a total amount emitted would be. As you may know, there is an ongoing campaign to establish 350 ppm as a desirable target. Such levels are political and can not be computed somehow. Even if we had decent values for the climate risks and their physical consequences, you’d need assumptions about long-term social and technological evolution. And you could not reason in monetary terms without assuming a discount rate and monetary values for the lives of unborn individuals or even for civilization itself. In the end, it boils down to how conservative you’re willing to be when faced with poorly understood long-term risks.
RE- Comment by Hank Roberts — 9 April 2010 @ 10:27 AM:
You are trying to deprive poor Gilles of his rambling and vacuous essay hobby. Shame on you.
Steve
AC # 115 : again, my posts here were only relevant to sea level rise. If the relaxation time is very long (many centuries, maybe 1000 yrs), all what you mentioned is mainly immaterial as long as the timescale involved is shorter. All the other points would deserve discussion, but it would be clearly OT and I would certainly be accused again of developing my .. ehhm. rambling and vacuous ideas. The only point I would stress is that on the century timescale, all fossil fuels will have been exhausted and that the “long term risks ” will probably have a totally different nature that what we can imagine now. Imagining the consequences of warming and sea level as if it would impact a society close to our current one is probably totally irrelevant.
Martin, Thank you for your #99 answering my #95.
Following up on that, it would seem that a much shorter smoothing time scale, than the 15 years that you use in modeling, would result in a significantly faster rise in sea level than the .08 cm/yr/deg that now is the outcome of model runs. Is there a way to know what it would be if the smoothing time scale was 3 years, for example?
As I understand what you are saying, this would also make the predicted temperature increase come out at a lower number.
Then it might make sense to discuss why the right number is 15 years.
Gilles (#117),
No, the timescale involved isn’t shorter. The timescale is in part exactly the same, for instance because the mixing rate of the ocean’s waters is a factor in the sea level as well as the atmospheric CO2 concentration.
Fossil fuels need not be depleted as fast as the current unsustainable consumption trajectory would imply. Your logic apparently goes like this: fossil fuels are going to be depleted soon anyway so there’s no reason to burn them slower. That’s circular logic.
Again, future temperatures and therefore future SLR will depend among other things on the rate of fossil fuel consumption. Just because some SLR is bad doesn’t mean more SLR isn’t worse. And just because much of the impact would take place over centuires doesn’t mean there will be no short-term impact. People are building and are planning to build infrastructure the usefulness of which will depend among other things on the rate of emissions in the coming decades.
To 90 (Efs_Junior) and 107 (Philip) Thanks for your comments. I have passed them on to my colleague.
Waqidi Falicoff 120: my comments got snipped partway through for some reason. This is a small fraction of the problems in the article you referred to. Your colleague should download the spreadsheet if he (or she) has any numeracy at all and will find that the whole thing is a total fraud: the formulae for the graphs on the site bear no relationship to the calculations in the spreadsheet.
AC : No, the timescale involved isn’t shorter. The timescale is in part exactly the same, for instance because the mixing rate of the ocean’s waters is a factor in the sea level as well as the atmospheric CO2 concentration.”
As I understood, mixing of layer is not enough to account for a very long time scale and other phenomena must be considered, e.g. the melting of land ice caps. And this cannot be stopped if the theory is correct.
“Your logic apparently goes like this: fossil fuels are going to be depleted soon anyway so there’s no reason to burn them slower. That’s circular logic.”
that’s not exactly what i’m saying : I think that the total amount of fossil fuels we can burn in the century will never reach this characteristic level (that I am asking you to ascertain) at which the cost of externalities will exceed the benefit they produce (which doesn’t mean of course that this cost doesn’t exist, but it will remain limited and manageable ). So actually it will never be interesting to reduce willingly the global amount we will burn. The rate at which they will be burnt is almost immaterial, but they will probably follow a bell shaped, Hubbert-like curve.
”
Again, future temperatures and therefore future SLR will depend among other things on the rate of fossil fuel consumption. Just because some SLR is bad doesn’t mean more SLR isn’t worse.”
Sure. I said that we can’t avoid several meters, but the choice would rather be between 10 m and 20 m if Stefan and Martin are right. So it could make sense to say “let’s reduce the amount of fossil fuel so that people in 1000 years have only 10 m instead of 20 meters”. Or it could also make sense to say “well the sea level will rise a lot anyway, so we’ll have to move away from the coast lines gradually, and the current cities on shore are doomed anyway. So it won’t cost a lot more to rebuild them farther inland. In any case, this will happen on a time longer that the life expectancy of what we build”. That’s an interesting debate.
#104 Lindsay, you might want to check my post on sea level variations on the Climate & Network connections.
Interesting article! (Loved the Freeman Dyson article, too.)
The most often-cited paper about sea levels these days seems to be Church & White (2006), A 20th century acceleration in global sea-level rise. They detected a 20th century acceleration in sea level rise of 0.008 ± 0.008 mm/yr^2 (and a larger acceleration if 19th century data was included). Although they noted that “no 20th century acceleration has previously been detected” by other researchers, their paper is widely referenced in support of the belief that the rate of sea level rise has accelerated in response to anthropogenic factors, especially the soaring CO2 emissions in the last half of the 20th century.
So what does that mean for sea levels in 2100?
Not much. Church & White now have newer data available (through 2007 instead of 2001), which shows considerably less acceleration than the previous data did. What’s more, despite their 2006 report of 20th century acceleration, there’s a very good reason to expect that the rate of sea level rise will not accelerate at 0.008 mm/yr^2 over the next 90 years. The reason is that (according to their latest data) it has not done so over the last 90 years.
In fact, when calculated using Church & White’s methodology and their latest data, the rate of sea level rise actually decelerated at 0.006 mm/yr^2 over the last 90 years for which they have data (1917 to 2007).
C&W’s methodology for calculating the acceleration is simple. They plot the GMSL (globally averaged, corrected & reconstructed mean sea level), and fit a quadratic curve to it via regression analysis. The acceleration is twice the quadratic coefficient.
Microsoft Excel can fit quadratics (Excel calls such fitted curves “trendlines”). So I downloaded C&W’s latest data, loaded it into Excel 2000, selected the last 90 years, generated an Excel X-Y “chart,” and fitted a quadratic to it. Here’s the graph:
http://i831.photobucket.com/albums/zz231/ncdave4life/church_white_1917-2007_trimmed-1.gif
And here’s the spreadsheet:
http://www.burtonsys.com/climate/church_white_2009_gmsl_90yr.xls
As you can see, the quadratic term is negative 0.0031, indicating deceleration of 0.0062 mm/yr^2.
Of course, -0.0062 mm/yr^2 is a very small deceleration. If continued for 90 years, it would amount to a decline in rate of global mean sea level rise of only about 0.56 mm/yr. (Likewise, C&W’s reported +0.008 mm/yr^2 20th century acceleration was also very small.)
Now, I’m not claiming that the rate of sea level rise is actually decelerating. It is possible to cherry-pick starting points to show either acceleration or deceleration of sea level rise during the last century, using Church & White’s data and method. For instance, using 1940 as the starting year shows deceleration, and using 1950 as the starting year shows acceleration. Likewise, choosing the last 100 years (1907 to 2007) yields a very small acceleration, and choosing the last 95 years (1912 to 2007) yields a very small deceleration (though in both cases the amount is so tiny that the quadratic curve appears to be perfectly straight).
The truth is that the tide gauge data for mean sea level for the last century is simply a straight line plus noise. There has been no measurable sustained acceleration or deceleration, regardless of whether you use the GLOSS-LTT tide gauges (individually or averaged), or Church & White’s latest data.
However, I have concerns about the reliability of C&W’s “corrected” data. It indicates a linear trend which is substantially higher than that which the GLOSS-LTT tide gauges show. The reason is probably given in this remarkable admission in paragraph 5 of their paper:
In other words, they added an ad hoc fudge factor! They didn’t directly say whether that “correction” in rate of GMSL rise was temporally uniform, but if so then it would affect only the linear trend, not the quadratic coefficient. So perhaps the acceleration/deceleration calculations are correct. Still, I’d like to know exactly how they processed the data before I completely trust it, even for calculating acceleration & deceleration.
Dave
Gilles (#122),
The melting of ice caps is a feedback as well (through albedo) which in turn affects other feedbacks including the melting itself. I hope you can see that the timescales actually overlap. Not that it matters much but that was your arugment.
You’re writing that the melting “cannot be stopped if the theory is correct”. But the theory is only about what would be likely to happen without intervention. Melting is maily a function of temperature and solar radiation. And aerosols could lower both and therefore halt the melting (at least if emissions were cut as well). You might argue that loading the startosphere with aerosols would be unwise but I don’t think you’d argue it can’t be done.
You wrote “on the century timescale, all fossil fuels will have been exhausted”. I’m glad you recognize now that this is not likely to be the case as a bell shaped curve of sorts is indeed expected (note that people also expect a long tail) but you keep clinging to your absurdly simplistic economics.
There is no “characterstic level” at which it would be rational to stop using fossil fuels. Would you have fossil fuels consumption continue without restriction until some day when it’s cut down to zero because some abstract threshold has been reached? How practical would that be? Please take a look at what’s actually being proposed: a gradual decrease in fossil fuel consumption.
Clearly, some of the consumption of fossil fuels today “produces” little to no benefit. The benefits/externalities line has been crossed generations ago by a growing share of the amount burned. This line goes across today’s consumption which is why people have been arguing that the consumption should be slowed and not either increased or cut to zero as your economics would have people believe is best.
Finally, do you have any idea of how the consequences of 10m and 20m of SLR differ? Surely you understand it’s not only a matter of rebuilding some cities (nevermind that the amount of rebuilding would vary).
Impressive story, and impressive study.
“refutations of silly null hypotheses” ,made me think about the long discussion on my blog, where eg the null hypothesis of extrapolating a linear trend was refuted. http://ourchangingclimate.wordpress.com/2010/03/01/global-average-temperature-increase-giss-hadcru-and-ncdc-compared/
Baron de Rais: Eat, drink, and be merry, for tomorrow we die.
Guillaume the Warmer: But your doing so at such a great rate is what will CAUSE us to die!
Baron de Rais: Are you trying to make everybody poor?
AC : I’m afraid you don’t really understand what a relaxation time scale is. If two time scales are involved, they don’t “overlap” : the longer wins over the shorter (at the longest time scale, all others seem to reach a quasi-equilibrium). Again referring to this figure https://www.realclimate.org/index.php/archives/2010/03/climate-change-commitments/#more-3070 , the characteristic time scale for temperature relaxation is not more than 100 years. If the relaxation of sea level with respect to temperature change is also of this order of magnitude, basically Stefan and Martin’s equation is not very accurate since a fair part of relaxation has already occured, and extrapolation at 100 years is not justified – actually we would be in an “intermediate” relaxation state where more accurate differential equation should be used. Their approximation of dL/dt propto (T-To) is admissible only if the timescale is much longer (they mention about 1000 years), in which case what I said applies : you can’t stop the rise for one millenium, whatever you do. In other words, either we can control rapidly the sea level rise, but in this case it reacts rapidly to temperature and the asymptotic level is not expected to be high (less than 1 meter as IPCC claims). Or the rise is on much longer timescale, it will be much larger, but we can’t act on it below century scale. There is no space (or very little may be for a very restricted narrow range of parameters and an very specific definition of the “dangerous” level and the “admissible” level) for “the rise will be very high if we don’t react rapidly” – although this may be the most popular slogan, it doesn’t correspond to any physical situation : if the rise threaten to be high, the system can’t react rapidly and if it reacts rapidly, it can’t be very high.
Gilles,
Your reasoning is too simplistic and abstract. Please keep the physical processes in mind. There are more than two time scales involved and, instead of “winning”, they feed back into each other. It follows that only the fastest processes will be allowed to reach equilibrium before the whole system does. There are much slower processes than ice sheet response or ocean mixing such as weathering by the way.
In the case of the sea level, it should be obvious that SLR would slow and that the sea level would be set on course for a lower equilibrium if temperatures dropped. No unphysical process would keep the SLR from decelerating (and eventually stopping if temperatures fall low enough). You can only use Rahmstorf & Vermeer to argue for an unavoidable centuries-long SLR by assuming that temperatures can not be brought down to begin with.
You are not linking to anything like the relaxation time scale for temperature (whatever that means physically). It looks like what you are linking to is basically ocean/atmosphere CO2 exchange combined with Charney feedbacks (but I may well be reading it wrong). In any case, the figure does not show how temperatures are thought to respond to a forcing. Instead, the forcing itself is simulated. As noted in the comments, the scenario simulated is a geoengineering scenario (although the authors apparently neglected to state it). In this scenario, you would definitely not have anything like 10m of SLR because temperatures would drop relatively fast. As it happens, this is the kind of scenario I had in mind in my last reply (#125) when I told you a large SLR was not a done deal.
If you want to look at the temperature relaxation time scale (although I think the concept is meaningless), I would suggest you look at the famous “Target atmospheric CO2” paper which talks about “several millennia”.
The time scale of CO2 exchange between atmosphere and oceans is more relevant to long-term SLR in an emissions reduction scenario anyway.
In closing, the systems reacts rapidly as well as slowly. The slow response is the one liable to cause the most damage but the fast response is the one that is likely to dominate in the near future. This is why Rahmstorf & Vermeer use a “dual model” (though there are more than two distinct processes in reality).
I find Stefan’s argument (in the linked Nature article) that increasing air temperatures at the poles will allow increased contributions to sea level rise from ice sheet melting to compensate the loss of glacial melt water. I’ve not heard this argument before, though the data on increased surface melt at Greenland tends to support this. Could you elaborate on this one of these days?
Eric made a comment a while back that seemed to indicate that he didn’t think polar ice sheet melting would continue to accelerate. I think he was talking about bumping up against the limits of ice sheet dynamics discussed by Pfieffer (?).
G the warmer:”In the case of the sea level, it should be obvious that SLR would slow and that the sea level would be set on course for a lower equilibrium if temperatures dropped. No unphysical process would keep the SLR from decelerating (and eventually stopping if temperatures fall low enough). You can only use Rahmstorf & Vermeer to argue for an unavoidable centuries-long SLR by assuming that temperatures can not be brought down to begin with.”
I fully agree. But in which scenario would the temperature decrease and what would be its average value over one millenium ?
From Stefan’s Nature commentary: “But this view considers only surface mass balance, without taking account of the kind of rapid, nonlinear ice-flow changes that some glaciologists expect for the future.”
That would mean that, taking into the account the very good fit with past measurements, this semi-empirical approach could serve as a warning system for future changes: If future sea level rise starts to become significantly larger than predicted by such approaches, that could signal that such rapid, non-linear contributions (from destabilizing ice sheets) are increasing.
As the ocean temperatures have been flat since 2003, I am curious what is purported to be causing the planet’s ocean to currently rise at the rate you are suggesting.
(i.e. Sea level rises due to increase in water temperature, increase in ocean mass (more water), or due changes in ocean floor level (geological time period, not relevant for this discussion.)
A simple mass balance calculation shows there is not sufficient ice melting to support the sea level rise rate you are suggesting.
Can anyone answer this question?
Mass and volume contributions to twentieth-century
global sea level rise
“The rate of twentieth-century global sea level rise and its causes are the subjects of intense controversy1–7. Most direct estimates from tide gauges give 1.5–2.0 mm/yr, whereas indirect estimates based on the two processes responsible for global sea level rise, namely mass and volume change, fall far below this range. Estimates of the volume increase due to ocean warming give a rate of about 0.5mmyr21 (ref. 8) and the rate due to mass increase, primarily from the melting of continental ice, is thought to be even smaller. Therefore, either the tide gauge estimates are too high, as has been suggested recently, or one (or both) of the mass and volume estimates is too low.”
http://www.grdl.noaa.gov/SAT/pubs/papers/2004nature.pdf
http://www.sciencemag.org/cgi/content/figsonly/327/5967/860
“Sea-Level Highstand 81,000 Years Ago in Mallorca
Global sea level and Earth’s climate are closely linked. Using speleothem encrustations from coastal caves on the island of Mallorca, we determined that western Mediterranean relative sea level was ~1 meter above modern sea level ~81,000 years ago during marine isotope stage (MIS) 5a. Although our findings seemingly conflict with the eustatic sea-level curve of far-field sites, they corroborate an alternative view that MIS 5a was at least as ice-free as the present, and they challenge the prevailing view of MIS 5 sea-level history and certain facets of ice-age theory.”
Comment:
For those who are interested in scientific puzzles. Why sea level has risen and fallen in the past is curiously controversial.
In the past there has been very rapid rises and falls of sea level that are too rapid, to have been caused by changes in the ice sheet masses. (During the last glacial period.) It appears something cyclic is causing the sea level to rise and fall. (i.e. The mass of the ocean remains essentially the same. The rise and fall of the sea level in the past does not align with planetary temperature changes. It is too early. An example of the cyclic sea level rise is during the odd Heinrich events.)
[Response: First of all, would you people out there please just quit with the ‘temperatures have been flat since (pick a date that works). Trends shorter than 15 years are pretty much meaningless. Why don’t people get this? Second the paper you cite does not conclude that there is any kind of mystery. They are simply saying that the various estimates of sea level rise, ocean expansion due to temperature, and ice mass loss are all uncertain, and it would be nice to be able to close the budget. Their conclusion is that the “mass increase plays a larger role than ocean warming in twentieth-century global sea level rise.” In other words, more ice has been lost than we thought, but ocean warming is still part of the picture. As for Heinrich events, yes, there is evidence for rapid changes in sea level not due to temperature, and the changes are so massive that glaciologists find it a bit startling that so much ice can calve from ice sheets into the sea in such a short time. But that’s what happened, and certainly indicates we have work to do in better understanding how ice sheets work. But the Heinrich events are ‘cyclic’ and have nothing to do with current sea level rise.(Which raises another question: where do people get the idea that saying “it must be a cycle” provides an explanation of anything?)–eric]
Thank-you for your comment.
The relative unexplained ocean level change in current times seems to be small however it is significant in terms of the necessary mass balance change or ocean temperature change that would be required to cause the ocean level to change the purported amount. A simplified calculation shows the current ocean level change cannot be explained by ice sheets melting or by ocean temperature change.
The Heinrich ocean level change was a fall and rise of ocean level of 10m to 15m which is easier to see as a puzzle. It is not possible for the ice sheets to loss and gain sufficient mass (melt and then gain mass due to snow fall)to cause the ocean level to fall and then rise 10m to 15m in the Heinrich time period (Roughly 1000 years.) As it is physically not possible for the ice sheets to lose (the planet does not warm up sufficiently during the Heinrich event time period, there is no physical mechanism that can move ice sheets off of the continents). It seems there must therefore be a third parameter that is causing the ocean level to change.
My point is a cyclic event (Heinrich changes including ocean level changes) requires a cyclic forcing function and that the forcing function must explain what is occurring during the event.
http://geochemistry.usask.ca/bill/courses/International%20Field%20Studies/Sea%20level.pdf
“Sea Level Change Through the Last Glacial Cycle
Furthermore, the pre-LGM period is characterized by substantial fluctuations in sea level of 10 to 15 m about every 6000 years. The timing of these rapid change events during oxygen isotope stage 3 (OIS–3) apparently coincides with Heinrich ice-rafting events recorded in North Atlantic sediments (61), which suggests that they reflect major ice discharges from continent-based or shelf grounded ice sheets (62).”
Gilles,
The scenario you linked to in #128 is one such scenario but it’s not realistic.
A halfway-realistic scenario in which temperatures decrease soon enough to mitigate a large SLR would in my opinion involve a combination of the following:
1) emissions reductions
2) CO2 sequestration
3) geoengineering
I don’t think one of these on its own would realistically be enough with current technology unless perhaps extreme measures are taken. For instance I don’t think emissions can be reduced to anywhere close to zero in the forseeable future.
As to the average temperature over a millenium in such a scenario, it would of course depend on the particulars of the scenario but there are also considerable uncertainties as to the workings of the climate system as you must be aware. I’m not qualified to propose realistic numbers but professionals have done so for other scenarios and I think we can extrapolate from that. I refer you to IPCC AR4 WG1, 10.7 for instance.
Irrespective of the uncertainties, it should be obvious that, if an effective geoengineering (and/or GHG sequestration) program aimed at controlling the SLR among other things ever gets started, a higher temperature peak would imply a slower return to the target temperature and therefore a much higher unavoidable SLR. This is why substantial emissions reductions in the next decades would leave more options open to future generations.
The inline rebuttals are excellent, but (sigh) it doesn’t seem to stop the stuff coming back.
I’m starting to wish y’all could apply a strikeout or light gray font color or something to flag the bogus rebunking claims when they reappear.
Arguing with repeated pasting of the same bunk never seems to help.
William points to a link to an online copy of a 2001 Lambeck and Chappell paper after claiming it’s impossible to explain changes of sea level happening in 1000 years, then argues some unknown effect must be hiding.
What does that paper actually say?
“… the rate of melting has been variable: In two periods of rapid and sustained sea level rise from about 16,000 to 12,500 and again from 11,500 to 8000 years ago, the rates of equivalent sea level rise approached 15 m in 1000 years (16, 17, 31)….”
and then discusses
“… substantial fluctuations in sea level of 10 to 15 m about every 6000 years. The timing of these rapid change events during oxygen isotope stage 3 (OIS–3) apparently coincides with Heinrich ice-rafting events recorded in North Atlantic sediments (61), which suggests that they reflect major ice discharges from continent-based or shelf-grounded ice sheets (62).”
That paper doesn’t say the change is impossible; it discusses changes and the uncertainties in what we know about both ice and land.
It doesn’t say there are gaps that require some unknown additional forcing to explain them, however.
Look at the reference at the original site, here:
http://www.sciencemag.org/cgi/content/abstract/292/5517/679
“Observations of glacially induced sea level changes also provide information on the response of the mantle to surface loading”
Look at the citing papers from the link there as well.
Then click the links for citing papers to see subsequent work in the area.
Accordings to Matthews (2009) (http://www.nature.com/nature/journal/v459/n7248/full/nature08047.html),
the effect of carbon emissions is proportional to the cumulative emissions and “the total allowable emissions for climate stabilization do not depend on the timing of the enmissions”. I read the paper a while ago and as far as I remember it validates Gilles’ argument that all that matters (on a timescale of decades to centuries) is the sum total of emissions and slowing down will have no discernible effect on the temperature reached at the end. Also even if Gilles is not right on the relaxation time (Martin says it’s far from clear whether it’s as bad as he proposes), he is of course right in saying that sea level will continue rising for some centuries even after an emission stop (see Plattner 2008, Long-term climate commitments). Also, as Martin rightly said in reply to Gilles, if we reach 2 degrees K (the climate target of many countries), we will be in sea level trouble. In my view it’s rather probable that with 2 K we will get the same sea level as during the last interglacial with the same temperatures (eg ca 6 m higher).
So in many ways, even though Gilles may be a bit repetetive, his arguments are not spurious and should be treated with due respect and seriousness.
In reply to comment #137 Hank Roberts
Hank,
What are you suggesting caused the sea level to change 10m to 15m every roughly 6000 years coinciding with the Heinrich events?
During the Heinrich events the sea level rises and then falls 10m to 15m.
If the entire Greenland Ice Sheet were to melt (2.85 million km of ice) global sea levels would rise 7.2 m (23.6 ft.) (IPCC 2001).
Why are sea levels currently rising? Mass or temperature?
Hank Roberts says: 11 April 2010 at 11:37 AM
I’m starting to wish y’all could apply a strikeout or light gray font color or something to flag the bogus rebunking claims when they reappear.
I heartily agree with this. There really ought to be a way of letting folks express themselves here yet at the same time providing some sort of early warning about the relative worth or worthlessness of an assertion.
On the thread concerning Der Spiegel, RC is making an admirable attempt to reset the tone of conversation on the site. Though I’ve thrown many logs on the fire of outrage myself, it’s a necessary change. Yet it is a sad fact that some people undeniably (heh!) use this site to propagate frankly incorrect and misleading talking points such as “the Arctic ice has recovered.” Hearing these repeated ad nauseam only to be laboriously deconstructed over and over again obviously taxes the patience of even the most saintly (such as Hank), inevitably inviting a breakdown in comportment.
Since all posts are scanned by actual people here prior to posting, perhaps the most rank and decayed wrong assertions could be highlighted? A simple change, really, perhaps a useful middle way.
Another tweak would be simply to set a budget on comments by an single person during a given period of time. As I understand the original intent of the site was to help people understand the science of climate change as opposed to simply being a venue for argument, but on the other hand arguments do unveil lots of interesting information.
If all this is too off-topic please feel free to delete…
Martin & Stefan:
Beautiful, concise and limpid paper. Congratulations! The results reflect the fact that, since the heating constant of the ocean is so much greater than that of the atmosphere, we can clearly see the effects of climate change on sea levels because the signal is unencumbered by the noise caused by atmospheric weather. Adding data from man-made reservoirs and introducing a time-lag constant that accounts for a decade-long mixing time of the top 100 m of the ocean is simply a stroke of genius and advances our understanding of hydrosphere behavior caused by global temperature variations. Accurate and precise prediction of the 1815 Tambora eruption is deeply satisfying, if not stunning. The model reflects reality. Period. End of story. The rhetoric stops here!
Well done!
Denys F. Leclerc, Ph.D.
C. Streif (#138),
This contrarian letter in Nature seems to have been written by the same Matthews who apparently neglected to take aerosols into account in his recent letter to Nature (see Gilles’ link). I would caution against giving it too much weight without giving careful consideration to the framework and assumptions that underly such statements as “the warming per unit CO2 emitted does not depend on the background CO2 concentration”. I would certainly have to reconsider my assertion that the effect of emissions is dependent on their timing if it was indeed the case that Matthews had overturned the conventional relationship between CO2 atmospheric concentration and warming.
As to Plattner, you may notice odd similarities between that paper and the section of AR4 I just referenced. You may also notice thats it is at odds with your Matthews quote (both can not be right).
Please note also that Gilles actually agrees with me about SLR: it will depend on the actual temperature curve and not on arbitrary assumptions or models. Models generally assume inaction (and rightly so). But to use this assumption in an argument against action amounts to circular reasoning.
[Response: Did he really say “the warming per unit CO2 emitted does not depend on the background CO2 concentration”?!? This is totally wrong!–eric]
> William
> Why are sea levels rising?
http://nsidc.org/sotc/sea_level.html
http://www.google.com/search?q=site%3Arealclimate.org+Why+are+sea+levels+currently+rising%3F
> C. Strief
> the effect of carbon emissions is proportional to the cumulative emissions
> and “the total allowable emissions for climate stabilization do not
> depend on the timing of the enmissions”.
Look more carefully at the Supplementary Information. They’re not talking about “the effect” — on the world and the biosphere. Neither is Gille
They’re talking about a particular measure useful to compare models:
“… The climate-carbon response (CCR) … aggregates both climate sensitivity…, a newly-defined carbon sensitivity (the airborne fraction of cumulative carbon emissions), and the effect of carbon cycle feedbacks on both the airborne fraction of emission and the resulting climate change. We have additionally shown that the CCR is approximately independent of both CO2 concentration and its rate of change ….
That ignores, for example, ocean pH — increasing very fast. Natural cycling is currently handling about half the fossil-fuel CO2 we put into the atmosphere. The leftover is what’s increasing in the atmosphere and ocean.
Cut back burning the fossil fuel far enough and the ocean pH quits changing.
https://www.realclimate.org/index.php/archives/2005/07/the-acid-ocean-the-other-problem-with-cosub2sub-emission/
https://www.realclimate.org/index.php/archives/2007/11/is-the-ocean-carbon-sink-sinking/
Can you possibly imagine that makes no difference in the outcome?
Eric,
Yes, that’s what he wrote but I have quoted him out of context, just like C. Streif did. The full letter with the footnotes should explain it but it’s beyond a paywall. I suppose the idea is that carbon cycle feedbacks would compensate for the lower forcing per mole as the concentration rises although it’s odd that they would compensate exactly. Presumably this relationship only holds within fairly narrow parameters, if certain assumptions are made and so on.
Eric: Google finds (if I can paste the working search string, let’s see)
http://www.google.com/search?hl=en&q=%22warming+per+unit+CO2+emitted%22
what’s probably the same text as the published paper:
http://www.cccma.ec.gc.ca/papers/ngillett/PDFS/nature08047.pdf
They’re talking about the ‘global warming potential’ of CO2 over time compared to other molecules, and how that’s affected over time (in ways different for CO2 than other molecules) by band-filling (radiation physics) and by ocean solution (physical chemistry) of that particular molecule.
This isn’t about temperature.
That claim is cited to their fn2:
2. Caldeira, K. & Kasting, J. F. Insensitivity of global warming potentials to carbon dioxide emissions scenarios. Nature 366, 251–253 (1993).
The abstract of that paper says in part:
“… for non-steady-state conditions, the integrated climate forcing from a CO2 perturbation depends both on the initial conditions and on future atmospheric CO2 concentrations. As atmospheric CO2 concentrations increase, the radiative forcing per unit CO2 emitted will become smaller because the strongest absorption bands will already be saturated. At the same time, higher concentrations of dissolved carbon in the surface ocean will reduce the ocean’s ability to absorb excess CO2 from the atmosphere. Each of these effects taken alone would affect the climate forcing from a pulse of emitted CO2 by a factor of three or more; but here we show that, taken together, they compensate for each other. The net result is that the global warming potential of CO2 relative to other radiatively active trace gases is nearly independent of the CO2 emission scenario. Thus, the concept of the global warming potential remains useful, despite the nonlinearities in the climate system and uncertainties in future emissions.”
—–
Please correct me if I’ve misread this; I’m just reading and moving my lips.
Hank #143
You mean ocean pH is dropping very fast, not increasing, right? was 8.3, is now 8.0-7.9; on a log scale, this is a huge change.
re: 146
A pH of 7.9 is predicted by the end of the century.
Re #133 William and response by eric,
It seems like there is a failure in the discussion process where sea surface temperature is confused with ocean heat content. Ocean heat content is of course an integral of temperature over depth, and of course the surface temperature might not go up much while deeper temperatures do.
To say that there is more sea ice lost as an explanation of sea level rise is an inadequately supported conclusion.
Back a few months ago we had a chart from NOAA showing a very significant increase in ocean heat content (measured only down to 700 meters) along with a suggestion of a plateau in global surface temperatures. (As I understand the climate modelers boundary conditions, global surface temperatures are the same as ocean surface temperatures, and the top “mixed layer” rapidly adjusts to that same value.)
My sense of things is that there is something wrong with the way the “mixed layer” is used to characterize vertical mixing of heat into the ocean. It looks like the ocean agrees with me, as represented by the actual NOAA data on heat content.
I have to acknowledge that I can not keep up with it all, but I have not found an analysis that relates NOAA heat content data to sea level rise. This would be a meaningful basis on which to discuss why sea level rise numbers are what they are.
@Martin (or Stefan):
Any interest in defending your paper @klimazwiebel? It seems there is some valid, but also markedly less valid discussion going on there:
http://klimazwiebel.blogspot.com/2010/04/sea-level-rice.html
Dear Martin and Stefan,
somehow I keep wondering about the difference between the here used smoothed data and the data from Holgate 2007)
It seems to me that for example if you would add the measured unsmoothed “Rate of sea level change” in the very first diagram of yours,
that there are periods where the measured data (including error bars) is far outside the range which is the basis of your calculations (for example around 1980 the rate drops below zero including error bars)
Are you aware, that oversmoothed data produces wrong trends.
All the best,
LoN