RealClimate

10 April 2007

Learning from a simple model

Filed under:

— gavin @ 7:59 AM

A lot of what gets discussed here in relation to the greenhouse effect is relatively simple, and yet can be confusing to the lay reader. A useful way of demonstrating that simplicity is to use a stripped down mathematical model that is complex enough to include some interesting physics, but simple enough so that you can just write down the answer. This is the staple of most textbooks on the subject, but there are questions that arise in discussions here that don't ever get addressed in most textbooks. Yet simple models can be useful there too.

I'll try and cover a few 'greenhouse' issues that come up in multiple contexts in the climate debate. Why does 'radiative forcing' work as method for comparing different physical impacts on the climate, and why you can't calculate climate sensitivity just by looking at the surface energy budget. There will be mathematics, but hopefully it won't be too painful.

So how simple can you make a model that contains the basic greenhouse physics? Pretty simple actually. You need to account for the solar radiation coming in (including the impact of albedo), the longwave radiation coming from the surface (which depends on the temperature) and some absorption/radiation (the 'emissivity') of longwave radiation in the atmosphere (the basic greenhouse effect). Optionally, you can increase the realism by adding feedbacks (allowing the absorption or albedo to depend on temperature), and other processes - like convection - that link the surface and atmosphere more closely than radiation does. You can skip directly to the bottom-line points if you don't want to see the gory details.

The Greenhouse Effect

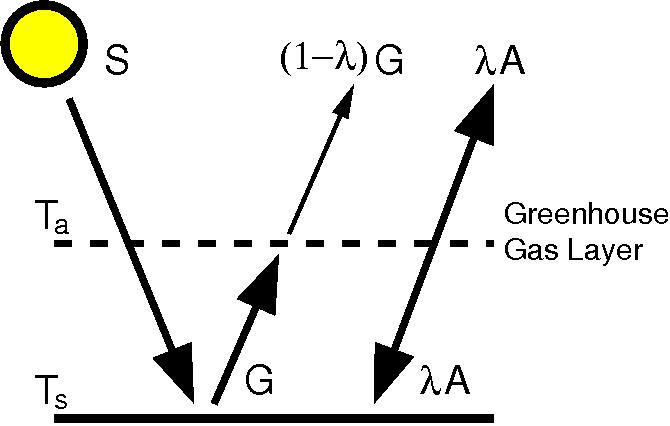
The basic case is set up like so: Solar radiation coming in is $S=(1-a) \mbox{TSI}/4$ , where is the albedo, TSI the solar 'constant' and the factor 4 deals with the geometry (the ratio of the area of the disk to the area of the sphere). The surface emission is $G=\sigma T_{s}^{4}$ where $\sigma$ is the Stefan-Boltzmann constant, and T_s is the surface temperature and the atmospheric radiative flux is written $\lambda A=\lambda \sigma T_{a}^{4}$ , where $\lambda$ is the emissivity - effectively the strength of the greenhouse effect. Note that this is just going to be a qualitative description and can't be used to quantitatively estimate the real world values.

There are three equations that define this system - the energy balance at the surface, in the atmosphere and for the planet as a whole (only two of which are independent). We can write the equations in terms of the energy fluxes (instead of the temperatures) since it makes the algebra a little clearer.

Surface: $S + \lambda A = G$
Atmosphere: $\lambda G = 2 \lambda A$
Planet: $S = \lambda A + (1-\lambda) G$

The factor of two for A (the radiation emitted from the atmosphere) comes in because the atmosphere radiates both up and down. From those equations you can derive the surface temperature as a function of the incoming solar and the atmospheric emissivity as:

$G=\sigma T_s^4= {S\over(1 - 0.5\lambda) }$

If you want to put some vaguely realistic numbers to it, then with S=240 W/m² and $\lambda$ =0.769, you get a ground temperature of 288 K - roughly corresponding to Earth. So far, so good.

Point 1: It's easy to see that the G (and hence T_s ) increases from S to 2S as the emissivity goes from 0 (no greenhouse effect) to 1 (maximum greenhouse effect) i.e. increasing the greenhouse effect warms the surface.

This is an extremely robust result, and indeed has been known for over a century. One little subtlety, note that the atmospheric temperature is cooler than the surface - this is fundamental to there being a greenhouse effect at all. In this example it's cooler because of the radiative balance, while in the real world it's cooler because of adiabatic expansion (air cools as it expands under lower pressure) modified by convection.

Radiative Forcing

Now what happens if something changes - say the solar input increases, or the emissivity changes? It's easy enough to put in the new values and see what happens - and this will define the sensitivity of system. We can also calculate the instantaneous change in the energy balance at the top of the atmosphere as $\lambda$ or changes while keeping the temperatures the same. This is the famed 'radiative forcing' you've heard so much about. That change (+ve going down) is:

$F_{Top}= \Delta S + \Delta \lambda (G_0 - A_0) = \Delta S + {{0.5 \Delta \lambda S } \over { (1-0.5\lambda) }}$

where $\Delta S, \Delta \lambda$ are the small changes in solar and change in emissivity respectively. The subscripts indicate the previous equilibrium values We can calculate the resulting change in G as:

$\Delta G \sim {\Delta S \over { (1-0.5\lambda) }} + {0.5 S \Delta \lambda \over { (1-0.5\lambda)^2 }} ={ F_{Top}\over { (1-0.5\lambda)}}$

so there is a direct linear connection between the radiative forcing and the resulting temperature change. In more complex systems the radiative forcing is a more tightly defined concept (the stratosphere or presence of convection make it a little more complex), but the principle remains the same:

Point 2: Radiative forcing - whether from the sun or from greenhouse gases - has pretty much the same effect regardless of how it comes about.

Climate Sensitivity

The ratio of $\Delta G/F_{Top}$ is the sensitivity of to the forcing for this (simplified) system. To get the sensitivity of the temperature (which is the more usual definition of climate sensitivity, $\Delta T_s/F_{Top}$ ), you need to multiply by ${0.25\over\sigma T_s^3}$ i.e. ${0.25\over\sigma T_s^3 (1 - 0.5\lambda) }$ . For the numbers given above, it would be about 0.3 C/(W/m²). Again, I should stress that this is not an estimate for the real Earth!

As an aside, there have been a few claims (notably from Steve Milloy or Sherwood Idso) that you can estimate climate sensitivity by dividing the change in temperature due to the greenhouse effect by the downwelling longwave radiation. This is not even close, as you can see by working it through here. The effect on due to the greenhouse effect (i.e. the difference between having $\lambda=0$ and its actual value) is ${ 0.5\lambda S\over(1 - 0.5\lambda) }$ , and the downward longwave radiation is just $\lambda A$ , and dividing one by the other simply gives $\lambda$ - which is not the same as the correct expression above - in this case implying around 0.2 C/(W/m2) - and indeed is always smaller. That might explain it's appeal of course (and we haven't even thought about feedbacks yet…).

Point 3: Climate sensitivity is a precisely defined quantity - you can't get it just by dividing an energy flux by any old temperature.

Feedbacks

Now we can make the model a little more realistic by adding in 'feedbacks' or amplifying factors. In this simple system, there are two possible mechanism - a feedback on the emissivity or on the albedo. For instance, making the emissivity a function of temperature is analogous to the water vapour feedback in the real world and making the albedo a function of temperature could be analogous to the ice-albedo or cloud-cover feedbacks. We can incorporate the first kind of physics by making $\lambda=f(T_s)$ dependent on the temperature (or for arithmetical convenience). Indeed, if we take a special linear form for the temperature dependence and write:

$\lambda (G) =\lambda_0 + \lambda^\prime ({G\over G_0}-1)$

then the result we had before is still a solution (i.e. $\lambda_0=0.769, G_0={S\over (1-0.5\lambda_0)}=390$ ). However, the sensitivity to changes (whether in the greenhouse effect or solar input) will be different and will depend on $\lambda^\prime$ . The new sensitivity will be given by

$\Delta G \sim { F_{Top}\over { (1-0.5(\lambda_0+\lambda^\prime))}}$

So if $\lambda^\prime$ is positive, there will be an amplification of any particular change, if it's negative, a dampening i.e. if water vapour increases with temperature that that will increase the greenhouse effect and cause additional warming. For instance, $\lambda^\prime=0.1$ , then the sensitivity increases to 0.33 C/(W/m²). We could do a similar analysis with a feedback on albedo and get larger sensitivities if we wanted. However, regardless of the value of the feedbacks, the fluxes before any change will be the same and that leads to another important point:

Point 4: Climate sensitivity can only be determined from changes to the system, not from the climatological fluxes.

Summary

While this is just a simple model that is not really very Earth-like (no convection, no clouds, only a single layer etc.), it does illustrate some relevant points which are just as qualitatively true for GCMs and the real world. You should think of these kinds of exercises as simple flim-flam detectors - if someone tries to convince you that they can do a simple calculation and prove everyone else wrong, think about what the same calculation would be in this more straightforward system and see whether the idea holds up. If it does, it might work in the real world (no guarantee though) - but if it doesn't, then it's most probably garbage.

N.B. This is a more pedagogical and math-heavy article than most of the ones we post, and we aren't likely to switch over exclusively to this sort of thing. But let us know if you like it (or not) and we'll think about doing similar pieces on other key topics.

Share This Blog reactions

296 Responses to “Learning from a simple model”

Spencer Weart Says:
10 April 2007 at 8:36 AM
We have to be careful here. One problem in the debate is people (engineers for example) who understand just enough math to get into trouble. Models that leave out convection, for example, can give weird, runaway results, which at one early point even cast some doubt on the whole theory. See my historical essay on simple models, in particular this incident.

Case in point: the statement “Point 2: Radiative forcing - whether from the sun or from greenhouse gases - has pretty much the same effect regardless of how it comes about.” Maybe true for a globally average temperature, but solar forcing causes warming at all levels, whereas greenhouse gases cause warming at the surface and lower atmosphere but cooling in the stratosphere, above where the radiation is blocked. In fact that is what is happening, and this “signature” has been cited by review groups as important evidence that the current warming is due to gases and not solar activity.

Simple models are valuable “educational toys” (as one scientist called them) for experts who understand what the models can and can’t tell, but they are toys with sharp edges.

[Response: Your points are very well taken…. Thanks. -gavin]
__earth Says:
10 April 2007 at 8:40 AM
I must protest! You said simple!
NU Says:
10 April 2007 at 9:14 AM
I’d like to see more of these posts. It’s illuminating to see how things work in a concrete model that one can plug numbers into and play with.
mankoff Says:
10 April 2007 at 9:39 AM
EdGCM is another very simple model that people can learn with. It is a bit more complex than the few equations in this post, but based on them, and simpler than most other GCMs. We’ve taken the time to move what are normally hard-coded or binary inputs to simplex external text files, so people can plug and play with different numbers and examine results.
JG Says:
10 April 2007 at 9:41 AM
Thanks for the math. Because of the multitude of simple explanations I can find at your site, I’m now ready for more technical explanations like this one. (There’s no need to post my comment; I’m just responding to the question.)
DT Says:
10 April 2007 at 9:48 AM
There is nothing to be scared about equations. This type of simple and fundamental atmospheric physics is exactly what the general public needs to know in order to counteract the non-sense put out by so many climate skeptics. Bravo.
Seba Says:
10 April 2007 at 10:18 AM
I actually prefer to see some more math from you guys. It will give me more elements to “fight” those skeptics
PR Says:
10 April 2007 at 10:23 AM
Would have been interesting to see the saturation point of the carbon radiation in the equations, as they don’t re-emit to infinity - as might be suggested here. A doubling in PPM from 200 to 400 may double temperature increase(for instance), but an increase from 400 to 800 will be less than double due to this saturation limitation.

[Response: That is an issue at a significantly higher level than you can properly deal with this model. You could just think about the emissivity being a logarithmic function of CO2, but actually there is a limit here of 1 for lambda - in the real world there is no such limit (which is related to the vertical structure of the atmosphere, the spectral nature of the absorption and things like pressure broadening effects). Look at Venus for an example of extreme CO2 forcing…. The effect doesn’t saturate, it just slows. - gavin]
tamino Says:
10 April 2007 at 11:10 AM
Personally, I’m glad for the math. But then, I’m a mathematician.

I suggest against doing this all the time; you’ll alienate much of your readership. But the occasional indulgence for those of us who want a quantitative rather than qualitative exposition, is a “breath of fresh air.”

And by the way, I find the exposition very clear.
M Says:
10 April 2007 at 11:15 AM
psuedo code so people could try it out for themselves…or some sensible range for numbers. nice to see variations in the articles.
tamino Says:
10 April 2007 at 11:37 AM
Although I love math (and already said so), I’ve had a lot of experience discussing scientific topics with non-scientists, and it’s most assuredly true that as soon as you mention numbers, a lot of their eyes “glaze over,” and if you dare actually to write an equation, most of their stomachs turn.

So I suggest against using math in public discussions and personal debates, unless you know that the parties involved are OK with it. Nothing will turn people off faster than equations.

That aside, I’d love to see more posts with equations from RealClimate (but for the sake of the lay readership, not too many).
James Says:
10 April 2007 at 11:47 AM
Just one complaint: while I appreciate the math, and would like to see more, I’d appreciate it even more if I could actually see the equations, instead of a sprinkling of random dots. Not really your fault, since HTML is majorly defective when it comes to displaying math, but would it be possible to add links to a pdf and/or LaTeX file?

[Response: Which browser/platform are you using? the equations are based on Latex derived images. I can see them clearly in Firefox, but if this doesn’t work on other browsers we won’t use it again… - gavin]
Barton Paul Levenson Says:
10 April 2007 at 12:13 PM
It just occurred to me, rereading this, that you’ve got 0 emissivity for no greenhouse effect and 1 for maximum greenhouse effect. Shouldn’t it be the other way around? If nothing is emitted the greenhouse effect should be infinite. On the other hand, if it’s one, you’ve just got the Stefan-Boltzmann equation.

Are you using emissivity in a different sense from the epsilon in the SB relation? I’m probably misunderstanding something here, so please set me straight.

[Response: I’m talking about the atmospheric emissivity (which equals absorption). No absorption = no greenhouse effect. - gavin]
Skip Says:
10 April 2007 at 12:39 PM
From an anthropological standpoint, I am concerned that we humans are repeating the errors of our predecessors. As I understand it, at least eight civilizations have perished because they diverted too much of their resources to attempting to control the climate. Although primitive by modern standards, these resources included even human sacrifice to try to make it rain. They perished from not only crop failures, but also reduction of their populations below a critical mass.

The issue that I have not seen explained fully enough with respect to global climate change is the effect of the heat of the core of the earth. I can’t help but notice the recent reports of increases of tsunami, earthquakes, el nino events, and volcanic eruption. If memory serves, these are products of the cooling and shrinking processes of the earth. As the inner core of the earth is hotter than the surface of the sun and heat rises, it stands to reason that the rise in ocean temperatures and chemical content of the sea and air, as well as contributions from the outer core and the earth’s mantel may be attributable to these. I would expect that these different contributions would manifest themselves differently on the earth’s surface due to tectonic plate overlap, differing depths of these contributions and depth and size of the various fissures in the earth.

Does anyone know of scientific studies that address these potential aspects of global climate change?

I just don’t want our civilization to mis-allocate it’s resources and go belly-up as a result.

Thank you in advance for any and all information provided.
Hank Roberts Says:
10 April 2007 at 1:16 PM
>equations
Wait, check the _other_ browsers first.

This page shows only a few errors in the validator
http://validator.w3.org/

Those aren’t, I don’t think, related to the equations.

Problems viewing may not be browser/HTML either.

Check your browser menu for something like /View/Character Encoding settings at the home end, see what fonts your computer/browser settings show it’s currently using; try Unicode (UTF-8) and Reload.

Some people may need ASCII (is this still true for vision-impaired, using text-to-speech? Used to be true for text browsers, like Lynx).

You could offer a picture of the equations in a JPEG file and a link, so those who can’t see them onscreen can download and print them.

Please, please do keep this up. I always need help thinking about things mathematically.
Adrianne Says:
10 April 2007 at 1:37 PM
I like the post, allthough I had to read it several times to follow the arguments.

By defining a model, I guess you can say we will get to the point where we get to control the model and change it. This has infinite advantages, but I am afraid the model is so complex that it will be very hard to define it accurately. And this is because there are so many factors that influence the terms of the equations.

Still, defining models is a must. And if it were that easy, we would have been able to determine weather trends.
matt Says:
10 April 2007 at 1:58 PM
I think your site is a great benefit to clarifying issues around cc and maintains a high degree of objectivity and scientific clarity,so thanks for that.Over the last couple of years I have followed the development of the cc issue but have become suspicious about a possible ingredient within the observations that may have some bearing and does not really get considered.I suspect the reason for excluding this phenomenon may be to do with a general view of the physics as they stand ,I dont know but here goes.I believe it is the case that the core of the earth itself is getting warmer.If this is the case then the consequences will be feeding into and magnifying other anthropogenic changes as well as increasing the influence of positive feedbacks in the climate system.I would appreciate your views on this, regards matt fairs
John Ross Says:
10 April 2007 at 2:06 PM
Thanks for a really good site and informative debate.

I have a query which is taxing an engineering mind.

RC appears to be satisfied that CO2 resides in the atmosphere for about 200 years, and that CO2 concentration has not exceeded 300 ppm in the last 400 kyear. I also see references to the absence of CO2 spikes in the ice core records as evidence that large volcanoes have not been a significant source of CO2 in the past.

I read elsewhere that samples of air in the ice cores might have been smeared by physical processes in the ice. This could be local mixing during compression, and possibly upward migration of the less dense air bubbles, a phenomenon which must result in some local mixing. Without getting too far into these details, the net effect would be akin to time-averaging . Detail will have been lost as a result.

Let’s say the atmospheric response to a large influx of CO2 is roughly the same as the response of a first order differential equation. The 200 year CO2 residence time would then equate to a time constant of about 66 years (i.e. only 5% of CO2 will remain longer than 200 years).

I have read that the ice core records of T and CO2 look like a 500 to 1000 year moving average. If this is reasonable, the ice core would hide actual CO2 perturbations following an event such as a large volcano. Only a small percentage of the amplitude of sudden perturbations would be evident.

If there is a significant averaging effect in the ice core, we would need to look again at those peaks. Whenever the peaks are close to the 300 ppm level, averaging could be hiding shorter periods when the CO2 concentration exceeds 300 ppm.

Do you have any references to literature which addresses these issues?

My guess is that there should be no averaging effect greater than 33 years (i.e. about 20% attenuation of sudden perturbations).

[Response: The 200 year number is already a great simplification and you can’t model it as a simple diffusion process - there are too many different physical processes and they all have different time scales. See this comment for more details: http://www.realclimate.org/index.php/archives/2007/02/aerosols-the-last-frontier/#comment-26374 and post http://www.realclimate.org/index.php/archives/2006/11/how-much-co2-emission-is-too-much/ - gavin]
James Says:
10 April 2007 at 2:25 PM
Re seeing equations: I’m using Opera on Linux, FWIW, but the problem isn’t browser-related. It’s down to the fact that because HTML doesn’t know about math symbols, equations are commonly rendered into images. Your images are black text on a transparent background, no?

That’s fine if the reader is using a colored background, but since more than a few minutes of looking at a colored background gives me a nasty headache, I have my browsers (and everything else I use) set to display colored text on a nice, restful black background. Which unfortunately means that the equation images show nothing more than vague outlines where grey pixels were used for antialiasing. This isn’t something an HTML validator is going to catch, since the HTML is perfectly valid but produces an unviewable result.

One partial solution is to render the images with a colored rather than transparent background (as some sites do), in which case I’ll see a white block containing the equation. A link to pdf or LaTeX source, though, would guarantee readability - plus the latter makes it possible to copy equations at need

[Response: Hmm. We are just using a standard plugin for the LaTeX rendering (latexrender). If you know of a fix or tweak to adjust the rendered images to have a white background instead of transparent, let me know. - gavin]
Barton Paul Levenson Says:
10 April 2007 at 2:33 PM
[[The issue that I have not seen explained fully enough with respect to global climate change is the effect of the heat of the core of the earth.]]

That’s because it’s trivial. The Earth system absorbs an average of 240 watts per square meter of sunlight. The geothermal flux averages 0.090 watts per square meter. Divide A by B.

[[ I can’t help but notice the recent reports of increases of tsunami, earthquakes, el nino events, and volcanic eruption. If memory serves, these are products of the cooling and shrinking processes of the earth.]]

Earthquakes are due to plate tectonics, which are driven by convection in the mantle, so to that extent you’re right. El Nino events are climate rather than geology.

[[ As the inner core of the earth is hotter than the surface of the sun ]]

It isn’t. The Earth’s core is at something like 3000 K, whereas the sun’s surface is 5779 K (and its core is at about 16 million K).

[[and heat rises, it stands to reason that the rise in ocean temperatures and chemical content of the sea and air, as well as contributions from the outer core and the earth’s mantel may be attributable to these.]]

No, the effect on temperature is trivial.
The Wonderer Says:
10 April 2007 at 3:06 PM
Re #1: What’s with the attitude towards engineers? This is the second such comment I’ve seen on this blog in the last few weeks. Is this a bitter attitude over past encounters, or professional snobbery? With an MSEE degree, I’ve had more than just enough math to be dangerous. And having spent significant time developing and using electromagnetic simulation programs, I’m plenty sympathetic towards the plight of the climate modeler. Feedbacks are a rather pedestrian concept in my field, and in any case, I didn’t find the post terribly difficult to follow.
Apart from the moderately contemptuous comments, I find your site fascinating and informative. For now, I’ll ignore those comments and just assume it must have been a result of unpleasant encounters with engineers from the lesser disciplines
jae Says:
10 April 2007 at 3:59 PM
Can anyone here explain why average July temperatures at low-elevation, extremely dry areas in the Desert Southwest are 3-4 degrees C higher than temperatures at the same latitudes east of the Rocky Mountains, which have extremely high absolute humidity? Both areas have the same top-of-atmosphere solar radiation, virtually same elevation. There appears to be some type of negative feedback in the east. Is it the influence of the Gulf of Mexico? Is it a negative water vapor feedback?
Arthur Smith Says:
10 April 2007 at 4:05 PM
Great to see this math - what I’d really like to see is a clear explanation of what it is about the real Earth that makes the sensitivity number several times as large as this simple model gives you. Aside from the factors you mention, there’s also the issue of pole-to-equator and summer/winter temperature variations that this simple zero-dimensional model misses (and I guess this is related to convection issues), but from the simple analysis I’ve tried that only adds a fraction to the sensitivity number. I’ve read a lot of the history on Dr. Weart’s site, excellent history by the way, but I find it very hard to track down a good physical explanation for what bumps up the sensitivity so much in the real climate system.
Steven H Johnson Says:
10 April 2007 at 4:09 PM
I recently prepared a short set of high level slides on global warming for my Rotary Club.

One of the concepts I drew upon was radiative forcing, using info on NOAA’s web page, http://www.esrl.noaa.gov/gmd/aggi/.

I did two calculations that were pretty interesting. Total solar energy reaching the earth on a daily basis. Total radiative forcing on a daily basis. The results were interesting, but also unsettling.

For the first calculation, net solar energy, I used the following. Energy per square meter - 235 watts. Diameter - 7914 miles (averaging polar and equatorial diameters). Result? Solar energy at 2,875,000 million terawatt-hours/day. An impressively big number.

For the second calculation, CO2 radiative forcing, I began with a value of about 1.66 watts/meter squared. I extrapolated to the planet as a whole. Result? 20,300 terawatt-hours/day.

I found the comparison unsettling. 20,300 terawatt-hours/day, sustained over a year, is a pretty big number, well over two full days worth of solar energy. Intuitively it feels like the sort of energy stream that’d heat the planet pretty darn fast, much faster than anyone is now seeing.

This leaves me wondering if I should have taken a different approach.

Should I have used a delta? Let’s say the Year 2007 begins at 382 PPM for CO2 and ends at 384 PPM. Using NOAA’s calculation approach, that’d give a Year 2007 delta of 0.028 watts/meter squared, or, planet-wide, a Year 2007 delta of 341 terawatt-hours/day.

Hansen and others have said that new increments of CO2 take 20 to 30 years to get fully absorbed by the oceans, the land, the air. 341 terawatt-hours day - if absorbed over 20 years at a linear amount - would translate into 1,250,000 terawatt-hours added to the heat of the planet. Equivalent to a shade less than half a day’s solar energy.

Intuitively, this approach feels much more reasonable. Is this how I should think about radiative forcing? As a year-by-year delta? This year’s new CO2 becomes this year’s new radiative increment, an addition to the heat of the planet that may take 20 to 30 years to be fully absorbed? Thanks for any insight you can give.
Arthur Smith Says:
10 April 2007 at 4:13 PM
On #22 - isn’t this a simple consequence of the high heat capacity of water relative to typical surface materials (i.e. rocks)? If there’s a lot of water around, it takes a lot more energy to raise the temperature than if it’s very dry. The low heat capacity of dry regions means they also lose heat a lot more easily, so their temperatures will tend to be lower at night and in winter. Anybody who’s lived near a coastline knows of the moderating influence on local climate.

The water vapor feedback discussed here is more of a net effect and much smaller than local daily or annual temperature variations: averaged over the year, does more water in the air tend to make things slightly warmer or not. Water is a known greenhouse gas, and night clouds retain heat, so it seems pretty clear the feedback is positive. There are many previous discussions of this effect on realclimate.
David B. Benson Says:
10 April 2007 at 4:14 PM
Gavin — This is well done! I encourage more similar snippets, maybe once each six weeks or so…
Hank Roberts Says:
10 April 2007 at 4:21 PM
>deserts, hotter

Speculating: less water vapor in the air by which actual incoming infrared from sunlight is being intercepted, so more direct heating of the ground by that band. And as noted, no significant water or water-containing organic material on the surface.

“Stay in the shade during the day. Sit on something 12 or more inches off the ground, if possible. DO NOT SIT ON THE GROUND as it can be 30 degrees
hotter than a foot above the ground…..” — common desert emergency info
James Says:
10 April 2007 at 4:48 PM
Re #14: [I can’t help but notice the recent reports of increases of tsunami, earthquakes, el nino events, and volcanic eruption.]

I think this one is easily explained: just rearrange “reports of increases” to “increased reports of”. These events aren’t (AFAIK, anyway) any more common than before; it’s just that, as with so much else, an omnipresent media reports on every isolated incident, and often enough inflates the reports to justify the coverage.
jae Says:
10 April 2007 at 4:52 PM
25: Yes, the increased water vapor in the east holds more heat and lessens diurnal variability, but this extra heat is not being registered as higher temperatures. Therefore, is the water vapor exerting a negative impact on surface temperature?
jae Says:
10 April 2007 at 4:56 PM
My math is getting a little rusty, along with my old brain. Can someone show how the three equations under the “Greenhouse Effect” section can be used to derive the equation for “surface temperature as a function of the incoming solar (radiation) and the atmospheric emissivity?”
Steve Horstmeyer Says:
10 April 2007 at 5:19 PM
On #22 There are several effects that make desert environments hotter than an equivalent environment laden with moisture: 1.soil moisture slows the warming of the soil and therefore the subsequent conduction of thermal energy to the atmosphere in contact with it as the soil moisture evaporates, 2. Moist areas usually have more vegetation, vegetation transpires moisture, which takes energy and absorbs solar radiation, some of which is used for photosynthesis and therefore also not available to heat the atmosphere, 3. A humid atmosphere is usually more turbid than a dry atmosphere. In this case I am referring to relative humidity, not absolute humidity, with less thermal energy available in the humid environment to do the work of evaporation (or alternately to keep the vapor in the gasseous phase)other materials in the air readily absorb moisture. These materials are called hygroscopic (sea salt, nitrate fetilizer dust, etc.)and are partly responsible for the formation of the haze in the “lazy, hazy days of summer”. The haze is the subject of “global dimming” of the sun, which by the way may be responsible for an underestimate of the amount of global warming, 4. Humid atmospheres may have more cumulus cloud cover.
This is not comprehensive but gives an idea of the many factors involved.

As far as mathematics, explanations and the public are concerned, generally forget it, I prefer to rely on concepts, analogies and simple explanations (i.e. very general or stripped of complications). It is amazing how the public misses the beauty and elegance of simple mathematics and has a panic attack at the very mention of an equation.
Marcus L. Says:
10 April 2007 at 5:36 PM
I like this post. It may be hard on some readers (although the above comments are encouraging), but it’s great for us sciency people who haven’t had the opportunity to take a college course in climatology.
tamino Says:
10 April 2007 at 6:04 PM
Re: #30 (jae)

Divide equation 2 by (2*lambda), you get A = 0.5 * G

Substitute (0.5 * G) for A in equation 1, you get S + 0.5 * lambda * G = G

Rearrange that, you get S = (1 - 0.5 * lambda) * G

Divide by (1 - 0.5 * lambda), you get G = S / [1 - 0.5 * lambda]

Voila!
DeWitt Payne Says:
10 April 2007 at 6:50 PM
This would have saved me a lot of time and searching a few weeks ago. I’ve already managed to drag myself up to this level and need more. Could you expand this with hyperlinks to more detail/complexity?

#31 Isn’t it actually the other way around? Ground water should speed the transfer of heat from the surface to the atmosphere which keeps the surface temperature from rising. Look at track temperatures at car races for example (or parking lots or sandy beaches). When the sun is out the (dry) track temperature is always much higher than the air temperature.
jae Says:
10 April 2007 at 6:51 PM
33: Thanks!
Hank Roberts Says:
10 April 2007 at 7:00 PM
Jae, think about nighttime in the desert. What happens then?
Chris C Says:
10 April 2007 at 7:08 PM
I liked this article. A few comments.

A few of the assumptions in this model should have been more clearly stated. Eg.

1. It was assumed that the atmopshere is transparent to incident solar radiation (why the S term did not appear in the Atmospheric radiative balence equation)

2. A single layer atmosphere with no lapse rate was used.

and the like. I thought the article was very well written, but I had to rely on my own knowledge of the subject to know what assumptions were begin made and how the model would deviate from reatlity.

I would love to see more articles of a similar nature however. Good work!
Jim Says:
10 April 2007 at 7:35 PM
I second that motion, whats with the attitude for engineers? I also have a MSEE and can’t quite get the attitude as we don’t get a primer for math we get the full treatment!
I appreciate posts such as these as well and had no trouble following it. You did well using simple math so the details don’t bog down what you are trying to say. I use IE7 and the equations looked fine. Is more stuff like this going to be forthcoming? (Since this is your day job! )

Jim
Patrick Kennedy Says:
10 April 2007 at 7:55 PM
I liked the post and the math is fine.

Can anyone point me to a quantitative explanation, at about the same level of detail as this post, of the relationship between emissions and atmospheric concentrations of greenhouse gases?
John Mashey Says:
10 April 2007 at 8:21 PM
(in general, nice math, please do it some more, but consider suggestion at the end):

#31: re math & public … it could be worse, and maybe it’s getting better…

Amusement, then serious point.
Amusement:
During the late 1990s, I had occasion to visit the US Congress’s computer people, who were proud of their efforts to get Congress to use computers & Microsoft tools. I naively asked:
so, what do Senators really use? do they do email? use Word? Use Excel? PowerPoint?

They laughed: “Are you kidding, they’re mostly lawyers, they like words a lot, but numbers and graphs?? forget it.” They said there were a few who could use such tools. I suspect it should be better now.

Seriously: making math accessible to a broader audience:

I sometimes ask:
for numerical calculations, what “programming language” is used by the most people?
people answer: FORTRAN? C? C++? Visual Basic? Java? Mathematica?

but I claim the likeliest answer is: Excel (or equivalents)

After all, people who do not think of themselves as progrmmers routinely do calculations with sometimes-nontrivial equations, that years ago would have required BASIC or FORTRAN coding. These days, Excel is often taught in middle-schools.
Google: excel equations middle school spreadsheets : 242K hits

For better or worse, many people who will go blank at classical math equations with lambdas and sigmas and such … could be handed the equivalent equations in an Excel spreadsheet [especially with a few embedded graphs], and would feel quite comfortable, and could easily play with the data.

SUGGESTION: like it or not, a lot more people read/write Excel than feel good with classical mathematical and notation, and the following can be really valuable, if the goal is to communicate the math more broadly:

a) Show Excel formulae as well as standard math notation.
b) Better, have a repository for simple, well-commented spreadsheets that can be downloaded and played with.

In addition, it becomes much easier to build on what’s there, given the wealth of builtin functions that one would never expect a person to use by hand, especially if examples ever want to get into simple statistical analysis.

This is *not* to suggest that standard math notation should be abandoned, or that Excel is the end-all tool (shudder), just that if one wants to communicate math broadly, it may well be that optimal pedagogy is in a state of rapid change, and many more people now “speak” Excel fluently than standard math notation….
David B. Benson Says:
10 April 2007 at 8:29 PM
Re #39: Patrick Kennedy — I went web trawling on the search phrase carbon cycle to find many excellent sites. Is this what you wanted?
Justin Wood Says:
10 April 2007 at 8:58 PM
Personally I think the maths is extremely welcome; it gives a fresh restatement of what I’m attempting to learn formally. But I humbly submit that some well placed diagrams would be excellent aids to understanding as well. For example, a simple illustration showing that ‘the atmosphere radiates both up and down’.

[First ever comment, but I eagerly read everything posted here…]
Simon Donner Says:
10 April 2007 at 9:42 PM
Nice job. It’d be great to see more posts - and more of us science bloggers writing posts - like this. Though that may tempt “some” people to argue that we are not properly framing our information.
Marion Delgado Says:
10 April 2007 at 9:54 PM
This is the Internets, home of “cheat sheets”

What we REALLY need are cheat sheets, not on the whole global warming climate change thing but on simple subdivision.

e.g. cheat sheet on C02. Would show net contribution of C02 by oceans, show various sinks and sources.

Or a cheat sheet on greenhouse gases. how water vapor responds to temperature change.

Thinking you take a FAQ of typical objections or questions and responses and a couple of stylized diagrams and just titles of objections and questions with 1 or 2 sentence summaries of the answers, mayhaps a couple of equations.
Steve Says:
10 April 2007 at 10:19 PM
I’m not sure I picked up the distinction between atmospheric absorption and atmospheric emission (not an engineer). How do these two relate?
Tracy Platt Says:
10 April 2007 at 10:29 PM
It’s nice to see some reasonably sophisticated discussion of climate modeling. I would just note that once the feedbacks are introduced the system becomes useless as a predictive tool. See for example http://www.neutralclimate.com/?page_id=28 for some examples of the sensitivity of a coupled, non-linear, dynamic system. Sorry, more math at that page.

[Response:Not really. Because there are systems that are unpredciticable, it doesn”t follow that all systems are. Why does the climate cool systematically whenever there is a large volcanic eruption? Why are the ice age patterns so regular and related to orbital forcings? Why are the seasons so clear? If the whole thing was completely chaotic and unpredictable none of this would be observable. - gavin]
David Warkentin Says:
10 April 2007 at 10:36 PM
As another engineer with plenty of math background, I too appreciated this post and would like to see more along the same lines. (A few sketches indicating the direction of the various components of the energy balance might make it even clearer, though.)

One reference I’ve found to be accessible is Roland Stull’s “Meteorology for Scientists and Engineers”. His chapter on climate change has an analysis similar to Gavin’s, with more on water-vapor, cloud, and albedo feedbacks at a similar level of detail.
Jeff Says:
10 April 2007 at 11:38 PM
I apologize in advance — I haven’t figured out how to write equations in Latex. For those who know just a smidgeon about math, it might have been nice to mention that the factor comes from the derivative of the Stefan Boltzmann equation: $dT/dQ = (4T^3)^{-1}$

[Response: I edited your equations to work with latex. You simply enclose them in [ tex ] and [ / tex ] pairs (no spaces). - gavin]
Edward Greisch Says:
10 April 2007 at 11:55 PM
I can read the equations OK, but they don’t copy. I could take snapshots [shift-control-3] or I could copy the article into Word5 for Mac and then re-create the equations with equation editor. But #33’s method:
Divide equation 2 by (2*lambda), you get A = 0.5 * G
Substitute (0.5 * G) for A in equation 1, you get S + 0.5 * lambda * G = G
Rearrange that, you get S = (1 - 0.5 * lambda) * G
Divide by (1 - 0.5 * lambda), you get G = S / [1 - 0.5 * lambda]

works really well. Why not do the math that way since we can all read and copy it? I am using a 16 year old Mac with OS 9.1 and ie5. The machine cannot be upgraded further. To read Adobe Acrobat [no higher than version 4] I have to sneakernet to another ancient machine. The max sneakernet file size is 1.3 meg. I cannot translate acrobat to word. LaTeX is out of the range of possibility. Please use Tamino’s method of writing math.
Doug Watts Says:
11 April 2007 at 2:08 AM
This is an excellent addition to Real Climate, even if the math totally eludes me thus far. I will figure it out.

For those of you old folks who grew up in Massachusetts, you probably remember Channel 4 meteorologist Don Kent often mentioning radiational cooling as being the cause of very low temps. on clear winter nights in places like Athol, Mass. and Keene, New Hampshire. Don Kent often took time to explain exactly what radiational cooling was, and how a clear dry winter night, without water vapor or clouds in the sky, allowed more heat to leak back into space, thus radiational cooling. I remember this from when I was 7 years old.

Also the history material provided by Spencer Weart in Comment #1 is outstanding.

This site is an enormously valuable resource, which is why I cite and post it as often as possible.
Zane Lewis Says:
11 April 2007 at 2:19 AM
I enjoy the challenge of obtaining a more robust understanding. Most people some to this site for more in depth analysis so I expected some math earlier. I support similar posts - but not to many. I suggest that you have a section on your site for a mathematical analysis. In addition you should have some links to “atmospheric math/science for dummies” so a lay person such as myself could get an even deeper understanding. In time there would be interest for sporadic intermediate or higher level postings that could be heavily linked for a newbie to obtain the necessary knowledge to follow the argument. Keep up the great work.
Nick Says:
11 April 2007 at 5:07 AM
I would like to see one article in the future.

It is clear that different factors affect the climate.

For example, solar, C02, …

Given the data it is possible to build a model that shows the contribution of each of the factors to global temperature in a statistical way. This shows how important in each factor was in the historical climate record.

The factors of which I’m aware are solar, atmosphere constituents, volcanos, orbital mechanics.

They all contribute and in somes cases with lags.

The statistical model then shows which are significant and which aren’t.

Now you move forward to the industrial era and you can now show what man’s contribution is, and whether or not it is statistically significant.

You can also take the models, and see if they predict the historical record, and if they are statistically different.

Nick

[Response: Try: http://www.realclimate.org/index.php/archives/2005/05/planetary-energy-imbalance/ -gavin]
Fernando Magyar Says:
11 April 2007 at 6:12 AM
Bring on the math. As the lucky father of a mathematically gifted 12 year old I’ll have him explain it to me.
Seriously though, unfortunately, most people think like the infamous jornalist Richard Cohen who wrote the piece entitled “What Is the Value of Algebra?”

http://www.washingtonpost.com/wp-dyn/content/blog/2006/02/15/BL2006021501989.html
Peter Hearnden Says:
11 April 2007 at 6:34 AM
I’m not good at maths but the way to get better can’t be no maths.
FTG Says:
11 April 2007 at 6:50 AM
Gavin,
I know this is already a simple model, but I think it would be a great addition to post the diagram that always gets drawn with it. I can try to dig one up at work, but I believe that just sketching a couple of arrows and scanning the figure would really help to make this actually “simple.”

[Response: You are absolutely correct. A figure has now been added. - gavin]
Alastair McDonald Says:
11 April 2007 at 8:21 AM
Re #55 It seems that this is a well known model. Does it have a name, or is there another way to cite it?
Barton Paul Levenson Says:
11 April 2007 at 8:27 AM
[[I’m not sure I picked up the distinction between atmospheric absorption and atmospheric emission (not an engineer). How do these two relate? ]]

For an object in “local thermal equilibrium,” the emissivity equals the absorptivity (Kirchhoff’s Law). In English:

Assuming no chemical or nuclear change, light can only interact with a material object in one of three ways –

* absorption — the object can absorb the light, usually heating up.

* reflection — the object can bounce back or scatter the light.

* transmission — the object can let the light go right through it.

Expressed as decimal fractions, these three have to sum to 1:

A + R + T = 1

To pick an example, the Earth’s surface absorbs about 95% of incoming light in the visual range, reflects about 5%, and transmits none at all (it’s opaque).

Now, every object not at absolute zero radiates photons. The power (energy per unit time per unit surface area) radiated by an object is:

F = ε σ T⁴

where F is the output per unit area (F for “flux”), σ is the Stefan-Boltzmann constant (5.6704 x 10^-8 in the appropriate units in the SI), and T is the temperature (degrees Kelvin in the SI).

The quantity ε is the “emissivity.” This can range from 0 to 1 for any real object. A perfect radiator with ε = 1 is a “black body” or “black body radiator.” But in practice very few objects are perfect blackbodies, most have an emissivity between 0 and 1.

Under “local thermal equilibrium,” ε = A (Kirchhoff’s Law again).
KS Says:
11 April 2007 at 9:12 AM
Engineer’s know just enough to get in to trouble? I think the engineer’s have a better handle on it than the scientists who never took the really hard classes. We actually have to design things that work. This article should have contained some info. on the stefan boltzmann equation forcing more cooling with increase in surface temp. but didn’t. “cooler because of adiabatic expansion (air cools as it expands under lower pressure)” Please check the def. of adiabatic. You may have meant the right thing but said it in the wrong way.
tamino Says:
11 April 2007 at 9:36 AM
Re: #58 (KS)

The solution to engineer-bashing is not scientist-bashing. Let’s judge each argument on its merits, regardless of its origin.

As for “cooler because of adiabatic expansion (air cools as it expands under lower pressure) Please check the def. of adiabatic,” a parcel of air does indeed cool as it expands adiabatically. Adiabatic does not mean isothermal.
Harry Haymuss Says:
11 April 2007 at 9:42 AM
One has to wonder what kind of engineer is being talked about here with “just enough math to get in trouble”. Obviously not engineers who have taken thermodynamics, and have been talking about convection on this subject for a long time. Maybe software “engineers”? “Hot air rises” is not a new concept…
Harry Haymuss Says:
11 April 2007 at 9:49 AM
P.S. you’re letting your prejudice show:

“Now we can make the model a little more realistic by adding in ‘feedbacks’ or amplifying factors”

Feedbacks are also damping factors.

[Response: Hmmm. Who is lettering their prejudices show? Who ever said that an ‘amplifying factor’ cannot be a ‘negative feedback’ (i.e., a ‘damping factor’ depending on precisely which variables you are talking about). Consider the radiation of outgoing longwave radiation (OLR) to space. As you increase the surface temperature, you tend to increase the OLR, in proportion to the 4th power of the temperature in fact. Seems like an ‘amplifying factor’ to me. Of course, its obviously a ‘negative feedback’ as well (warmer surface temperatures leading to greater heat loss from the surface), in fact the key negative feedback that prevents runaway warming of the Earth’s surface over time. In other words, it is an ‘amplifying factor’ with respect to OLR, but a ‘damping factor’ with respect to surface warmth. - mike]
Dan Says:
11 April 2007 at 10:03 AM
re: 60. ??? Nothing in the original statement said ‘feedbacks’ could not be damping factors. It was “‘feedbacks’ OR amplifying factors” (emphasis added). To assume otherwise is showing your prejudice.
Steve Milesworthy Says:
11 April 2007 at 10:18 AM
Adding up the A1B scenario greenhouse gas forcings for 2100 taken from the IPCC TAR I get about 4.1 Watts/m^2, most of which (3.48) comes from CO2.

The calculation above gives a temperature rise of 1C relative to 2000, but the models (in the 4th assessment report SPM) give a range of about 1.8-4.2C rise for the A1B scenario which require upto 8-9 Watts more forcing.

So what are the other processes in the models that are causing the additional warming? Section 7.2.1.1 suggests that water vapour doubles (or more) the warming. Is this it? Or are there other major effects.
Barton Paul Levenson Says:
11 April 2007 at 11:57 AM
Steve –

Doubling CO₂ increases the Earth’s temperature 1.2 K by itself (Houghton 2004) but 2-4 K with all the feedbacks (IPCC 2001, 2007).
Isidore Says:
11 April 2007 at 12:08 PM
Thanks for this introduction to climate modeling.

A quantitative model is a quantitative hypothesis. We have observed historical climate data over the last 150 years which we can test against climate models/hypotheses. A climate model which ’simulates’ (fits) past observations better than another is more likely to be true. There are too many variables so some approximation is inevitable.

The climate debate is about whether there is a significant human cause of current global warming.
- Climate skeptics no longer doubt that the earth is warming, they claim the causes are purely natural eg sunspots and volcanoes.
- The IPCC does not doubt that there are natural causes of recent global warming but they claim 90% certainty that this warming has a significant human cause.

These competing hypotheses can be tested by quantitative models see UK Met Office Climate Change Myths.
http://www.metoffice.gov.uk/corporate/pressoffice/myths/index.html

The two graphs next to Myth 2 show that natural causes alone cannot explain the increase in earths temperature since about 1975. But If human factors are added to natural causes we get a much better fit to the observed data.

AFIK climate skeptics have not been able to provide a quantitative model of natural-only causes which fit the observed data so well. Please correct me if I’m wrong.

This is the key data the climate skeptics ignore, instead they attack the straw man that ALL climate change is man-made.
SebastianDell Says:
11 April 2007 at 12:36 PM
So, climate models do simply explain it all?

(Yes, I am a denialist, an skeptical, or whatever thing you call those people who does not trust the global warming as a matter of faith)
Harry Haymuss Says:
11 April 2007 at 12:44 PM
#62, The context is feedbacks (aka) amplifying factors, as RC typifies feedbacks as generally positive. This statement just entirely negates the potential for damping feedbacks - RE#64. Of course #64 discounts the fact we know next to nothing about cloud feedbacks - even the sign.

#65, don’t confuse CO2 forcings with all anthropogenic forcings, including land use changes, black carbon and other dust on ice, etc. Re those other changes, could not we tell the magnitude of the difference in CO2 forcing compared to some of the other anthropogenic forcings by looking at the temperature trend of the Arctic vs. Antarctica?
Steve Milesworthy Says:
11 April 2007 at 1:39 PM
#63 and #64 I know that water vapour is a feedback, not a forcing, but since water vapour seems to be so important I’m really looking for an addition to the simple model that says X amount of warming results in Y increase in water vapour, and barring clouds etc. this water vapour adds an additional “radiative forcing” of Z - such that a few iterative calculations can provide an estimate of the temperature rise.

If such a simple extension is not helpful, then why not?
Dan Says:
11 April 2007 at 1:57 PM
re: the parentheses in 66. Of course it is not a matter of faith. No one shoud accept it as that. It is a matter of science. Data, experiments, hypotheses, conclusions, peer review…the scientific method. It works. And the science behind global warming is quite strong.
Alastair McDonald Says:
11 April 2007 at 2:04 PM
Feedbacks come in two very different types. 1) Negative feedbacks which, despite their name, are good and stabilise the system by damping any changes. 2) Positive feedbacks which, unlike their name, are bad and amplify changes. Once they get over 40% they cause oscillations or strange attractors. If they get over 100% they produce a runaway effect - just like what happened in the rapid climate change at the end of the Younger Dryas stadial.

So you can have negative feedbacks and amplifying factors, or damping effects and positive feedbacks, but not feedbacks and amplifying factors. “Amplifying factors” is redundant when used that way.

HTH,

Cheers, Alastair.
Dan Says:
11 April 2007 at 2:10 PM
re: 67. No, not at all. RC does not typify feedbacks as generally positive. To assume so is a quite clear prejudice. As a simple example, a quick search on “negative feedback” at the top of the page yields, among other things, http://www.realclimate.org/index.php/archives/2006/08/climate-feedbacks/
Both positive and negative feedbacks are discussed in that one specific example. Now particular feedbacks may be discussed as positive, such as CO2 or water vapor, simply because they are.
Joe White Says:
11 April 2007 at 2:33 PM
Ian Plimer is again getting some mileage here in Australia. This ran as a lead story on news.com.au and has already been the subject of a couple of op-ed pieces here. Lots of tired old stuff that have been covered countless times before, yet for some reason, this fellow seems to attract more media than he deserves.

http://www.news.com.au/story/0,23599,21542564-2,00.html
EntropyFails Says:
11 April 2007 at 2:47 PM
I just wanted to say that I’m very glad to see this sort of article on your site. RealClimate is one of the most popular climate sites on the net so I think that you do your readership a great service by bringing them up to speed on the basics of climate science. The people who honestly want to learn about climate science understand that they need to learn the basic mathematics of the science.

I’d go even further and say that I think you should long running series of articles based on this simple model that eventually build up to as close of a model to the ones found in IPCC report as possible. Given the collective will of reasonable people to not kill life on earth, using your site to raise the cognitive models of how people view climate should help raise the level of debate due to people with good models being able to trash the arguments of people with bad models. You do that a lot here in your comment section, so I think by putting a series of articles together that can help others learn to trash similarly poor arguments they encounter in their own lives you give the truth a weapon.

In short, kudos and I hope this is not the last of the “more technical” articles. This site is your bully pulpit and I think your readership will appreciate any help in lifting their cognitive models.
Bill Says:
11 April 2007 at 3:11 PM
Thanks…now if these types of articles were just available as PDFs (or even PS files)….quibble, guibble…More of the same would be fine with me.
John Mashey Says:
11 April 2007 at 3:16 PM
re: #72:
As I recall, news.com.au belongs to Rupert Murdoch, i.e., like Fox News.
I conjecture that’s adequate reason for this to appear there.

I’ve been in Oz about dozen times, and unless it’s changed recently:
a) You have a *lot* of coastline, and most Australians live near it.
b) Most of the big cities aren’t very far above sea level. I’d be a little nervous for places like the Whitsundays, Freemantle, the Gold Coast, Cairns, even with 1-2-foot rise. Canberra seems safe.
c) You could use more water.
d) Like here in CA, you have trouble with forest fires, especially when it’s hot.

At least the article was a nice checklist of bad ideas
tamino Says:
11 April 2007 at 3:46 PM
Re: #73 (EntropyFails)

you should long running series of articles based on this simple model that eventually build up to as close of a model to the ones found in IPCC report as possible…

I’d love to see such a work. But it would involve much more complicated mathematics (vector calculus), and much more complicated physics (the Clausius-Clapeyron equation is not for everybody). So I don’t think it would be appropriate as a series of blog posts.

It would make an appropriate textbook. So if any of you RC guys have such a work, at that level, available as pdf, then … bring it on!
Jamie Says:
11 April 2007 at 4:04 PM
because I’m not sure where else to post this, is there any resource that I might use to explain climate anomalies such as the cold snap we are having in April, within the global warming debate?
MacDoc Says:
11 April 2007 at 4:58 PM
Gavin

You made mention of being careful about cold events in relation to climate change.

Strikes me with more energy in the system - gradients are steeper and boundary conditions between high and low pressure systems may vary from the longer term geographic norms…..ie stronger Pacific storms might force cold continental systems further south and east at times just as a for instance.

More energy retained I would think would produce more chaotic boundaries with higher energies, - an abnormal incursion of warm or cold air masses might push farther and run into geophysical regions ( ie Great Lakes or mountain funnels not reached in a less energetic atmosphere. )

Seems to me that gradients might build higher before releasing as well in an energetic/chaotic climate so while cold events might occur outside the norms from time to time as air masses collide with more energy.

I would also think the jetstreams would show increased energy levels which again might mean unusual cold intrusions.

I’d be happy to be set straight but seems to me that “outside the norm” excursions of cold air or water could be expected in some locales in an overall more energetic system.
Hank Roberts Says:
11 April 2007 at 5:48 PM
Jaime, someone (maybe you? I forget) asked that elsewhere recently. Weather isn’t climate, so this is the wrong website; have you tried NOAA?
http://www.cpc.ncep.noaa.gov/products/tanal/montoday/mon2day.gif
Hank Roberts Says:
11 April 2007 at 5:50 PM
One more for Jamie, this might be helpful (just found with a quick Google search, not something I can evaluate, you might find reading up on this a challenge worth taking on):
http://weatherclimatelink.blogspot.com/2007/01/no-fooling-around-possibly-severe-cold.html
Harry Haymuss Says:
11 April 2007 at 6:12 PM
Could not we tell the magnitude of the difference in CO2 forcing compared to some of the other anthropogenic forcings by looking at the temperature trend of the Arctic vs. Antarctica?

[Response: No. The impact of any forcings depends on a number of issues - local heat capacity (much higher in the Southern Hemisphere due to the greater extent of oceans), difference in dynamics and impacts of other factors (like the ozone hole). It’s therefore not as straightforward as one might hope. - gavin]
Joel Shore Says:
11 April 2007 at 9:22 PM
Re #77 (Jamie): The question is whether the cold snap that we are having here in the Eastern U.S. is really that unusual. Sure, it has been colder than average for several days…maybe even a couple standard deviations colder than average for a few of them. But, I didn’t get the impression it was record-setting cold. (A few places may have broken DAILY records…that is the record for a particular day…but those are relatively easy to break.)

The point is that in the absence of crunching through the statistics, one can’t really determine how anomalous this cold is. And, frankly, I don’t get the impression that it is that anomalous. It takes a lot of work to actually pull out a trend from a system with large fluctuations.
Steve Says:
11 April 2007 at 9:26 PM
Reference #57

First off, thanks for responding.

This “local thermal equilibrium” concept appears important.

I can understand the idea of solid objects radiating at each, and having the same radiation field if they are at the same T and are made of the same material. I think I have a reasonable grasp of black body radiation coming from objects based on the temperature of the object.

It makes sense that bodies of gases in the same state should have the same radiation properties.

In either case, the net radiation should be zero.

Black body to balck body, gas to gas.

You are losing me at the gas/solid interface. Does this concept of “local thermal equilibrium” apply to the gas/solid interface? Are there other concepts that are necessary to consider in this case?

If a gas is in contact with a black body, and they are both at the same temperature, does the concept imply that they will have similar radiation characteristics? I am not clear on this point.
Ike Solem Says:
11 April 2007 at 9:31 PM
These kind of posts are very valuable and much appreciated, even if they take much longer to read through.

Regarding “Point 1: It’s easy to see that the G (and hence T(s) ) increases from S to 2S as the emissivity goes from 0 (no greenhouse effect) to 1 (maximum greenhouse effect) i.e. increasing the greenhouse effect warms the surface.”, I’d suggest including a graphical representation of the equation, with lambda on the x-axis and both G and T(s) on the y-axis. A picture of an equation is a lot easier for the layperson to understand than an algebraic expression and helps remove the equation fear factor (and is also easier to see).

Also, simple terms like emissivity can be confusing, but wikipedia and other sites have good descriptions: http://en.wikipedia.org/wiki/Emissivity

As to how engineers can get into trouble with math, this is not really a dig at engineers - cross-field misunderstandings are many. Comments on positive and negative feedbacks by engineers whose experience is with electronics are a good example and have appeared on RC. Understanding feedback in an electronic circuit doesn’t mean that you understand the multi-variable and competing positive/negative feedbacks that affect climate, from albedo to water vapor to carbon cycle effects (once I spent five minutes talking to a history teacher about positive and negative feedbacks and climate before I realized he thought I was saying ‘good’ and ‘bad’ - whoops!)

Anyway, this kind of thing is very useful. A simple box model of the carbon cycle could be treated similarly.
Jamie Says:
11 April 2007 at 9:34 PM
Interesting. thanks for your responses. The type of people I am in conversation with at times use this as “evidence” of the “myth” of global warming.
Richard Ordway Says:
11 April 2007 at 10:01 PM
I think this post is great!

It clearly breaks down the basic “voodoo-witchery” mystery of how climate models work for the public.

This is something they can see, feel and manipulate…and dare I say relate to better now because of this post. Outstanding! Great topic!
Doug Watts Says:
11 April 2007 at 10:57 PM
As a friendly note to those who suggested Mr. Schmidt et al. should do “this” or “that” on this site. Do it yourself or give these folks a $100 or more donation.

I do find it funny about those who demand extreme levels of scientific proof that high amounts of CO2 can heat up a planet, given … hmmm … Venus.

It’s that neon-argon Venusian atmosphere, I guess.
wayne davidson Says:
11 April 2007 at 11:40 PM
#77 This Southern cold snap was one month in the making in the North American Arctic (during March), studied it with great fascination before it went to your location, it simply slowly moved South since early April. I would rather look at the rest of the world, as a sure sign of the summer weather to come, In its wake, Arctic temperatures made an about face, total rebound in temperatures, from record cold to record warm in a few days. I’ll have an optical breakdown of this March cold air mass on my website at the end of April, Density weighted temperature reached 231 Kelvin at its coldest point, it was like being in the reverse eye of an hurricane, no wind, extremely low tropopause and very little heat.

I appreciate this post, as there is not enough equations…. Examples about practical use of these equations would be greatly appreciated.
Robin Johnson Says:
12 April 2007 at 1:42 AM
RE #22:

In addition to good points made by Steve in post #31, I would add the following.

Some of the solar energy turns liquid water and soil moisture into water vapor instead of “heating” the surface and near-surface air. Instead of raising the temperature of the soil, it induces the phase change. The resulting water vapor is lofted upward (lower density than the “air”). The total heat in this case is the same - its just distributed differently making the effective air temperature at the surface cooler. In the desert, the heat is concentrated at the surface of the absorbing/reflecting object.

At night, in the desert, a significant fraction of the heat absorbed is quickly radiated away. In the wet environment, the additional water vapor created during the day absorbs the long wave radiation emitted at night (greenhouse effect) and then as the night wears on and the air cools, the water vapor condenses back to the surface warming the surface and near-surface air by giving “back” the heat required to become vapor. Of course, some of the water vapor lofts high into the atmosphere and flows away from the source redistributing that heat elsewhere (stupid complexity issues…)

The net effect being the desert is really hot during the day - but fairly cool at night. Over areas of high moisture (ocean, jungle, etc). The day is very warm (but typically under 40C) and humid, but the night is warm and humid. If the albedo effects are a “wash” and the convection doesn’t carry off too much vapor, the “average” temperature (24 hour day) is going to be higher in the wet climate than the desert climate at the same latitude. But you can die from heat stroke in both places.
pete Says:
12 April 2007 at 4:06 AM
I aint a scientist but id like to know what HAARP contributes to global warning if any?
Mark Ritzenhein Says:
12 April 2007 at 8:21 AM
Being a simpleton, and not having been forced to endure mathematics for a couple of decades, it would be helpful if you better explained all math symbols (particularly the subscripts and superscripts, Kelvin scale, the tilda, etc.) used (even if that bores you and all of the engineers), to plug in real figures, and to provide more explanation of the involved factors. I followed some of the equations but eventually gave up.
Don’t underestimate the ignorance of the lay person…
Barton Paul Levenson Says:
12 April 2007 at 8:58 AM
Here is Gavin’s model (without feedbacks) in the Just Basic programming language. Sorry for the way it appears in HTML, e.g. the apparent lack of indents. < pre > wasn’t much of an improvement. For anyone who wants to use it, just cut and paste into your own Basic interpreter or compiler, or translate to C or Fortran or whatever.

‘******
‘—–
‘ Gavin.bas implements a simple greenhouse model.
‘—–

sigma = 5.6704e-08 ‘ Stefan-Boltzmann constant.
answer$ = “Y” ‘ Response from user.

while answer$ = “Y”
input “Solar constant? Observed is 1367.6: =>”; S
input “Bond albedo? Observed is 0.306: =>”; A
input “Emissivity? Observed is 0.769: =>”; lambda

Ftop = (S / 4.0) * (1.0 - A) ‘ Find absorbed flux.
G = Ftop / (1.0 - 0.5 * lambda) ‘ Find ground emission.
Ts = (G / sigma) ^ 0.25 ‘ Find ground temperature.

Print
Print “Ftop =”, Ftop ‘ Display results.
Print “G =”, G
Print “Ts =”, Ts

Print
input “Again [Y/N]? =>”; answer$ ‘ Repeat if user wishes.
answer$ = upper$(answer$)
Print
wend
end
Lynn Vincentnathan Says:
12 April 2007 at 11:19 AM
RE “factor 4 deals with the geometry (the ratio of the area of the disk to the area of the sphere)”

I like to visualize things. Does this 1/4 have to do with the sun hitting a flat (if made small enough thru calculus) area on earth, as opposed to a spherical area. Or does it have to do with a flat area (if made small enough thru calculus) of the sun aimed at us, as opposed to the radiation from its entire spherical surface (i.e., we don’t get the whole of the sun’s radiation, only 1/4 — which seems a bit large, since we are so small)?

What is the Stefan-Boltzmann constant? Is that the temp of the earth, if you go down, say, 6 feet? I know there is a some constant of about 55 degrees F (assuming there isn’t a extreme deep freeze that bursts the pipes).

If so, I believe that’s the principle behind geothermal heating/cooling systems. If it’s 10 below zero F outside, you pump 55 degree air through the underground pipe system, then warm it a bit more to comfort level. I think it may also work as an AC as well.

What is “emissivity”? Is that like reflection of warmth, or reflection plus existing warmth (the earth’s constant being maintained in the process)? Or infrared waves?
Mike Donald Says:
12 April 2007 at 11:30 AM
Hi chaps,

How about sending this article to that Viscount Monckton? Not that I’m implying anything of course of someone who’s not afraid of sending in the lawyers…

http://environment.guardian.co.uk/climatechange/story/0,,2053520,00.html
Richard Ordway Says:
12 April 2007 at 11:39 AM
Here are some links, some of which have to do with models underestimating the rate of world-glacier/ice shelf disintigration.

The models don’t include yet (or weakly model) alot of the ice physics, such as positive feedbacks such as melt ponds, crevasse propagation, thermal ice diffusion, buttressing/ keystone effects, moulin, water lubrication effects ..remember Gavin’s feedback equations above!!!)…

and some mention of Gavin’s work too. Fascinating and understandable.

http://portland.indymedia.org/en/2007/04/357344.shtml
tamino Says:
12 April 2007 at 12:39 PM
Re: #94 (Lynn Vincentnathan)

RE “factor 4 deals with the geometry (the ratio of the area of the disk to the area of the sphere)”

I like to visualize things. Does this 1/4 have to do with the sun hitting a flat (if made small enough thru calculus) area on earth, as opposed to a spherical area. Or does it have to do with a flat area (if made small enough thru calculus) of the sun aimed at us, as opposed to the radiation from its entire spherical surface (i.e., we don’t get the whole of the sun’s radiation, only 1/4 — which seems a bit large, since we are so small)?

It has to do with the fact that the earth intercepts sunlight proportional to its cross-sectional area (pi * R^2), but that energy gets spread over the earth’s surface area (4 * pi * R^2). So the 1366 W/m^2 for a square meter directly facing the sun, is diluted by (p * R^2) / (4 * pi * R^2) = 1/4 of that, or about 341.5 W/m^2.

What is the Stefan-Boltzmann constant? Is that the temp of the earth, if you go down, say, 6 feet? I know there is a some constant of about 55 degrees F (assuming there isn’t a extreme deep freeze that bursts the pipes).

No, the Stefan-Boltzmann constant relates the energy emitted by a radiating object to its temperature. Warm objects (”warm” meaning above absolute zero) radiate away some of their energy as electromagnetic waves (light). The amount they radiate depends on temperature. Since energy is measured in one set of units (Joules, ergs, watt-hours, whatever) and temperature in another (deg.K), there has to be a conversion factor: the Stefan-Boltzman constant is it.

If so, I believe that’s the principle behind geothermal heating/cooling systems. If it’s 10 below zero F outside, you pump 55 degree air through the underground pipe system, then warm it a bit more to comfort level. I think it may also work as an AC as well.

The Stefan-Boltzman constant is unrelated to geothermal heating. But the earth, below its surface, is indeed hotter than the surface, and we can use that temperature difference to drive a heat engine, extracting the energy for useful work. Also, the earth’s subsurface temperature doesn’t follow the same annual cycle (seasons) that surface temperature does. And, the deeper you go into the earth, the hotter it is.

What is “emissivity”? Is that like reflection of warmth, or reflection plus existing warmth (the earth’s constant being maintained in the process)? Or infrared waves?

No, it’s the radiation of energy. The energy is already there, as heat (or other forms), but objects naturally tend to give that energy away as electromagnetic waves (light) — that’s emissivity.
Barton Paul Levenson Says:
12 April 2007 at 12:57 PM
[[I like to visualize things. Does this 1/4 have to do with the sun hitting a flat (if made small enough thru calculus) area on earth, as opposed to a spherical area. Or does it have to do with a flat area (if made small enough thru calculus) of the sun aimed at us, as opposed to the radiation from its entire spherical surface (i.e., we don’t get the whole of the sun’s radiation, only 1/4 — which seems a bit large, since we are so small)?]]

The Earth intercepts sunlight on its cross-sectional area, which is pi R^2, where R is the Earth’s radius. But its actual surface area is that of a sphere, 4 pi R^2. So the Solar constant has to be divided by four to see how much sunlight hits an average square meter of the Earth.

[[What is the Stefan-Boltzmann constant?]]

It’s the proportionality constant in the Stefan-Boltzmann radiation law:

F = $\epsilon\sigma$ T⁴

F is the flux the object radiates, power per unit area (watts per square meter in the SI). $\epsilon$ is the object’s emissivity (discussed below); $\sigma$ is the Stefan-Boltzmann constant, T is the temperature (degrees Kelvin in the SI).

The value of the Stefan-Boltzmann constant is about 5.6704 x 10^-8 W m^-2 K^-4 in the SI. In the English system it would have a different value and the units would be something like BTUs per second per square foot per Rankine degree to the fourth power.

[[What is “emissivity”?]]

It’s µ from the above equation, and it describes an object’s efficiency as a radiator. An emissivity of 0 means the object radiates nothing at all (it’s surrounded by some kind of perfect insulator). An emissivity of 1 means the object is a perfect or “black body” radiator. Most real objects have an emissivity somewhere in between.
Jeff Says:
12 April 2007 at 1:03 PM
Re 93 (Lynn’s comments)
One way to visualize the factor of four difference due to the geometry of a disk versus a sphere is to imagine a colander, which one might use to strain spaghetti, for example. The top surface, which is open, is shaped like a disk, while the bottom screened surface is shaped like a hemisphere. The same quantity (heat, light, electric field, etc.) that passes through the curved surface must pass through the disk-like surface, as long as no heat, light, or charge is generated in the colander itself. In other words, the flux passing through the curved surface must be the same as the flux passing through the disk. This is the essence of Gauss’s Law. When you calculate a flux, you have to take into account the angle between the field line and the normal to your surface. If the sun’s rays come in at a glancing angle, your flux is smaller. The flux is zero at the edges of the sunlit side of Earth, and it is a maximum at the center of the sunlit surface. Now you could systematically integrate across the hemispherical surface of Earth, taking into account the angle of the sun’s rays, or you could remember that the flux is the same for a disk or a hemisphere. The reason for this is because the sun’s rays are perpendicular to the disk everywhere.
As for your question about Stefan-Boltzmann’s constant, it might help to consult a reference such as Wikipedia to get a feel for what the relationship is. I have my students investigate the Stefan-Boltzmann law by using a light bulb connected to a variac, which is used to systematically change voltage. They have to connect an ammeter and a voltmeter, so that they can calculate the resistance of the tungsten filament as the bulb gets brighter and brighter. By examining published tables for resistivity of tungsten as a function of temperature, students can estimate the filament temperature at each voltage step. By plotting power (current times voltage) against temperature on a log-log plot, they get an exponent of about 3.9. My hope is that by using commonplace objects, students will realize that many objects obey Stefan-Boltzmann’s equation, not just distant stars like our Sun.
James Says:
12 April 2007 at 1:34 PM
Re #93: [If so, I believe that’s the principle behind geothermal heating/cooling systems. If it’s 10 below zero F outside, you pump 55 degree air through the underground pipe system, then warm it a bit more to comfort level. I think it may also work as an AC as well.]

Err… Not exactly. What you’re describing is ground source heating/cooling, though it’s often miscalled geothermal in ads. A true geothermal heating system uses heat from a hot spring or similar. A ground source system uses a heat pump to take advantage of the fact that the ground below the frost line stays at a fairly constant 55F or so year round. Thus in the winter, the system can actually move heat from the relatively warm ground to the house using less energy than it would to heat the cold outside air, while in summer the reverse is true.

If this seems like magic to you, well, it does to me too
Larry Risch Says:
12 April 2007 at 2:30 PM
Re:

The basic case is set up like so: Solar radiation coming in is S=(1-a) mbox{TSI}/4, where a is the albedo, TSI the solar ‘constant’ and the factor 4 deals with the geometry (the ratio of the area of the disk to the area of the sphere). The surface emission is G=sigma T_{s}^{4} where sigma is the Stefan-Boltzmann constant, and T_s is the surface temperature and the atmospheric radiative flux is written lambda A=lambda sigma T_{a}^{4}, where lambda is the emissivity - effectively the strength of the greenhouse effect. Note that this is just going to be a qualitative description and can’t be used to quantitatively estimate the real world value

It isn’t the math that gets me, when I was taught pi=C/D I was also told what circumference and a diameter was.

What is albedo, what is the disk(area of sunlight?) (is the sphere is earth?) , and Stefan-Boltzmann constant, and surface temperature and the atmospheric radiative flux
Robin Johnson Says:
12 April 2007 at 2:38 PM
Lynn-

The geometry of the sphere works like this. If we draw a circle around the Earth where light transitions to dark and then project an imaginary cylinder back to the Sun, we can measure the solar output striking the Earth. Even though the Earth is a bulge at the bottom of that cylinder - the amount of solar light hitting the Earth (at whatever angles) is just the cross section of the cylinder - which is exactly the area of that circle we drew - which is, of course, given by Ï� * r².

It turns out that the surface area of a sphere is given by the formula 4 * Ï� * r². Even though the light is only striking half the Earth, we really want to know the total energy distributed across the entire surface area of the Earth since the acquired heat is not instantaneously radiated back into space. Given that, we divide the area of the sphere by the circle, we get really simple ratio of 4 to 1. So, we have to divide the solar output per meter squared by 4 to get the actual solar output per square meter over the Earth.
DeWitt Payne Says:
12 April 2007 at 5:31 PM
[what is emissivity]

An emissivity of zero means the object in question is either a perfect reflector or is perfectly transparent and as a result does not absorb and hence cannot emit radiant energy and has nothing to do with being surrounded by insulation. Gold metallized plastic film is a near perfect reflector and is used on spacecraft to prevent radiant heating from sunlight. A planet with an atmosphere of a noble gas like argon would have no greenhouse effect because argon is transparent in both the visible and infra-red wavelength region. If you have an infra-red kitchen thermometer and don’t use the emissivity correction, a stainless steel pot full of boiling water will apear to be at a much lower temperature than a black anodized aluminum pot full of boiling water. Stainless steel is a good reflector and thus has a lower emissivity than black anodized aluminum.
DeWitt Payne Says:
12 April 2007 at 7:17 PM
If lambda can’t be greater than one, then isn’t the maximum surface temperature 303 K with this model, given a constant solar irradiance of 240 W/sq.m.? Yes, that’s fifteen degrees warmer than now, but it’s also an upper limit. I second the motion for something between this oversimplified model and a full bore planetary GCM. How about creating a multi-layer spreadsheet model or program of a one-dimensional, clear air, Earth normal nitrogen, oxygen and argon atmosphere containing only water vapor and carbon dioxide as ghg’s with graphs and charts of temperature and pressure with altitude. Of course, the more user adjustable parameters, the better. Including sensible and latent heat transfer from the surface and how they change with surface temperature and humidity would be nice too.
The Wonderer Says:
12 April 2007 at 7:24 PM
Re: #84: Ike,

I agree cross-field misunderstandings may be many and Iâ??m certainly not suggesting that engineers are all-knowledgeable or infallible, by any means. Climate science is certainly rich in underlying complexity, and I do not pretend to be an expert (unlike the engineer to whom you refer). Iâ??m just saying the misunderstandings are probably not due to the math itself. Linear algebra as used in this example should be in the basic toolkit of any engineer, and while climate feedbacks are multi-variable and non-linear, they are conceptually the same if not analogous to those found in other disciplines. Someone who believes that engineers â??know just enough math to get into troubleâ??, lives in the modern world, and is otherwise rational, should be very afraid to get out of bed in the morning. As far as history professors, wellâ?¦. theyâ??re interesting too.
Pat Says:
12 April 2007 at 9:55 PM
Here’s how I might try to qualitatively introduce radiation transfer in a more complete manner:

(LW = longwave = terrestrial = the kind of radiation emitted at typical atmospheric (except thermosphere, which, in terms of amount of energy, has a very small role to play in the totality of atmospheric radiation) and near-surface temperatures of the Earth - as opposed to SW = shortwave = solar (radiation))

(optical depth, optical thickness, optical length, optical distance* = a measure of path length relative to the rate of absorption+scattering along that path - an opaque object has infinite optical depth, a transparent object has zero optical depth; the proportion of light starting at a point and moving along a path of optical thickness t which is neither scattered nor absorbed is exp(-t)
* PS I’m assuming all these terms can be used, correct me if I’m wrong)

(To the degree that objects are exchanging LW radiation, the net heat transfer via radiation will be from hot to cold)

At any given level in the atmosphere (and at any given wavelength), radiation coming from any direction is originating from a distributed source along the pathlength, the distribution declining exponentially with optical distance; as the optical thickness increases along a given geometric distance, photons travel shorter paths, so the radiative energy exchange increases along shorter distances while decreasing along longer distances; hence, for LW radiation:

increasing LW opacity (from increasing greenhouse gas concentration, or from clouds)
=> increased cooling to space from those parts of the atmosphere optically closest to space, decreased cooling from the surface and, if the starting opacity is high enough, lower atmosphere, to space, and if the starting LW opacity is high enough, decreased cooling from the lowest parts of the atmosphere to the colder upper parts, although - depending on the temperture profile and other details (wavelength dependencies) - increased cooling from the very top of the troposphere and the upper stratosphere to the base of the stratopshere, etc.

Temperature rises or falls until, at any given level, emitted radiant power per unit atmosphere = absorbed radiant power per unit atmosphere + convergence of sensible heat transport by convection + net latent heat release per unit atmosphere

net latent heat release = ((condensation + freezing) - (evaporation + melting))
Harry Haymuss Says:
12 April 2007 at 10:09 PM
Re the response to #81, that sounds good until one remembers that Antarctica is over 13 million square km. Surely you’re not suggesting ghg warming of that much area is all damped by an ocean sometimes thousands of km away? Just doesn’t hold water…
Harry Haymuss Says:
12 April 2007 at 10:16 PM
Re #71, your example of water vapor being a positive feedback is unrealistic. Clouds are a product of water vapor, and it is not known if that feedback is positive or negative. To say “water vapor is a positive feedback” is academic - and I thought we were trying to address reality here.

You didn’t address #64, which states feedbacks *double or triple* the effect of CO2.
Francis Massen Says:
13 April 2007 at 2:52 AM
Concerning G: Am I right that the given expression assumes that the emissivity of the ground is 1? There was a presentation at Santa Fe (2006) by Hartwig Volz from RWE showing that neglecting the emissivity of the large ocean surfaces (which is lower than 1) leads to climate sensitivities that are too high, and that this correction is absent from all (?) climate models used by the IPCC. What does Gavin think about this?
BTW: I really appreciate some more theoretical contributions like this one. Please go on! Just a recommendation from someone who has taught physics since nearly 40 years: please don’t jump over intermediate calculating steps (like the derivative of G), short-cuts are a challenge for the reader but may put off someone used to more extended developments.

[Response: This derivation assumed an emissivity of 1 at the surface. In the real world it’s slightly less (0.95 or so depending on various conditions), but that just divides through to the sensitivity and actually makes it s